Periodic Table |
 |
 |
 |
 |
 |
 |
 |
| Chemistry & Complexity: Systems Thinking | Chemistry & Complexity: Non-Linear Systems |
Linear Chemistry Systems
The science of chemistry – chemistry, the whole thing – is analysed in terms of systems thinking over three pages of this web book. This second page deals with linear (predictable) chemistry systems.
The first question is:
- Why are there any linear chemistry systems at all? Why do gases behave in such a way that the Ideal Gas Equation, PV = nRT, is linear?
The Ergodic Hypothesis, The Principle of Equal a priori Probability & The Correspondence Principle
It is a fundamental assumption of statistical mechanics & kinetic theory that a closed system with many degrees of freedom, such as a gas in a closed container, ergodically samples all equal energy points in phase space.
The ergodic hypothesis [and here], states that the time averaged behaviour of microscopic quantities – atoms & molecules – gives the same result as the macroscopic "ensemble" average, where the "ensemble" is a collection of all the possible states that than an assembly of molecules would reach in an infinite amount of time.
In other words: When a chemical system is at equilibrium the time-average is equal to the ensemble-average.
- Due to the astonishing size of Avogadro's number, 6.022 x 1023, the techniques of statistical mechanics can be used to explain and derive chemical thermodynamic principles, and therefore understand the bulk behaviour of gases, liquids, solids, solutions & mixtures.
- Fluids are very complicated on the microscopic scale, however, the Navier-Stokes equations depend only upon the density and viscosity of the fluid to describe bulk behaviour. The chaos of the individual molecular trajectories disappears on large scales.
- For a step-by-step look at the philosophy and logic underpinning kinetic theory, look here.
The ergodic hypothesis was important in the 19th century, early in the development of statistical mechanics. These days the generally accepted principle of equal a priori probability [and here], states that for a quantum system the probability of an entity being found in any particular quantum state is equal to the probability of that entity being found in any other quantum state, with the constraint that the total energy must equal the sum of the energies of all the particles.
The principle of equal a priori probability is time independent and it can be used to derive the Boltzmann distribution. The implication is that the time dependent ergodic hypothesis is, strictly, not necessary. But, as discussed in this link, it is currently not possible to mathematically prove [in an elegant manner] the correctness of the principle of equal a priori probability.
The correspondence principle – first invoked by Niels Bohr in 1923 – states that the behaviour of a quantum mechanical system will reduce to classical physics when the quantum numbers are large. This means that: either some quantum numbers of the system are excited to a very large value or the system is described by a large set of quantum numbers, or both.
Conclusion:
The ergodic hypothesis, the principle of equal a priori probability and the correspondence principle "explain" (give a theoretical and philosophical underpinning and justification to) the ability to extrapolate from the world of microscopic chemical species and quantum mechanics – atoms, ions, molecules – to the macroscopic world of bulk substances and materials.
Indeed, classical physics "works" because simple physical "laws" emerge on large scales:
| Microscopic Entities |
→ | Materials |
| atoms, ions & molecules | gases, liquids, solids |
Dilute, Homogeneous, Ideal Solutions
Linear behaviour is generally observed in homogeneous [stirred] solutions with concentrations ranging from 0.1 mol per litre (mol dm–3) or less to "infinite" dilution (but not past the Avogadro dilution limit where statistically no analyte species are present).
Ideal dilute solutions are associated with:
Henry's law, Debye-Hückel theory, the Beer-Lambert law, the osmotic law, the Nernst equation, etc.
Acidity (pH) and the solubility of salts in the presence of common ions can be predicted.
Many analytical techniques exploit the linear, predictable behaviour of the dilute solution regime. The procedures are surprisingly general:
- First a representative set of samples for analysis are obtained.
- If the samples are solids, such as rock, pharmaceutical tablets or biological specimens, the samples are ground to a fine powder or otherwise homogenised.
- The analyte species are dissolved and/or extracted into a suitable solvent, the choice of which is often crucial.
- The dilute analyte solutions are filtered and assayed by one of many techniques: titration, optical absorption, electrochemical potential difference, NMR, etc. If the analyte is a mixture, the analysis may be preceded by chromatography step.
- The assay results are compared against a calibration curve, which should be a straight line, prepared from samples of the same analyte and of known concentrations:
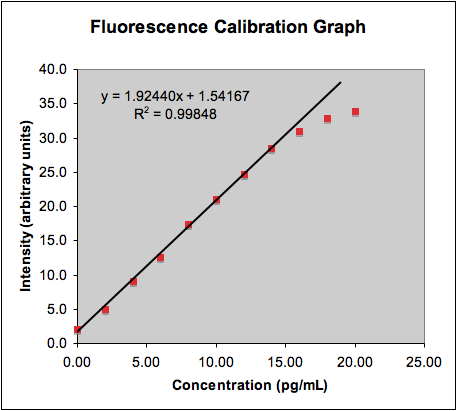
- Note that non-linear effects can arise from limitations in the analytical technique which are independent of the linearity of the ideal dilute solution. For example, pH probes behave poorly in alkaline solutions and UV/vis optical adsorption detectors are susceptible to stray light and other errors.
The question of where dilute, homogeneous solutions can be found in chemistry space is addressed towards the end of this web page, here.
Conclusion:
Linear phenomena are commonly found in dilute homogeneous solutions. It follows that when looking for linear behaviour, experiments should be conducted in the dilute homogeneous solution regime whenever possible.
Equilibrium Thermodynamics
Chemists are familiar with idea of thermodynamic "open systems" and "closed systems", where (for example):
A jar half-filled with solvent and with the top on is a closed system, but when the top is removed the system becomes open and the solvent is free to evaporate.
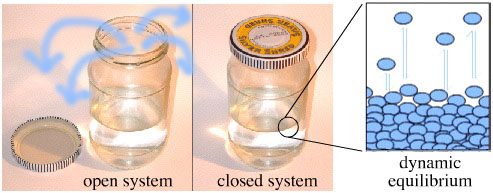
The liquid-gas phase boundary is seething with activity: at the surface of the liquid individual solvent molecules continuously leave and enter the gas phase, before and rejoining the bulk liquid. In the closed jar this activity is at dynamic equilibrium with equal numbers of molecules moving in each direction. In the open jar, more solvent molecules enter the gas phase and then leave the confines of the jar than rejoin the liquid phase.
- Only closed systems can be perfect and completely uncomplicated.
- Only closed systems can exhibit dynamic equilibrium.
- Open systems can never be at true equilibrium, although careful experimental design may approximate to closed. For example, we can measure the pH of a 0.0100 molar acetic acid solution in an open flask because the time constant for acid-base equilibrium is many orders of magnitude faster than evaporation of water and acetic acid from the flask.
- Only mathematicians and physical scientists have access to true closed systems. All biological systems are open. (The closest exception to this statement is the amazing Ecosphere, but even the Ecosphere requires light.)
- Generally, chemical systems are dynamic, even though they may appear static to the untrained eye.
Equilibrium chemistry involves understanding how chemically dynamic systems behave when a parameter: temperature, pressure, concentration or catalyst, is changed. For example, what will happen to the equilibrium position of a particular gas phase reaction if the pressure is increased while the temperature remains constant.
With a profound understanding of matter, heat and energy, a group of 19th century scientists – including Clausius, Helmholtz, Gibbs, Maxwell, Carnot, Thompson (Lord Kelvin) & Boltzmann – developed mathematical models: the first, second and third laws of thermodynamics and kinetic theory, that describe how chemical systems equilibrate.
So successful was this approach that Einstein was later to say: "thermodynamics is the only physical theory of universal of content I am convinced will never be overthrown".
Theoretical ideas in thermodynamics are readily transferred to engineering. A classic and historically important example of this technology push is the Haber-Bosch process for the synthesis of ammonia, NH3. This was the first large scale industrial chemical reaction process to be performed under high pressure conditions:
N2 + 3H2 ⇆ 2NH3
Qualitative predications in equilibrium thermodynamics space can be made using Le Châtelier's principle, a very useful "trick" for making predictions about the law of mass action.
Likewise, the phase rule can be used to predict how gases, liquids and solids coexist and equilibrate in phase space.
On the subject of phase, students of chemistry often think (and are taught) that "physical" phase change processes like melting, boiling and sublimation, are somehow different from "chemical reactions", like the reduction of benzene to cyclohexane.
However as shown in the equations below, both the evaporation of water and the catalytic hydrogenation of benzene to cyclohexane can be described by chemical equations that are identical in form: both reaction systems can be balanced in terms of mass, enthalpy & entropy and Gibbs free energy, so they are far more similar than they are different:
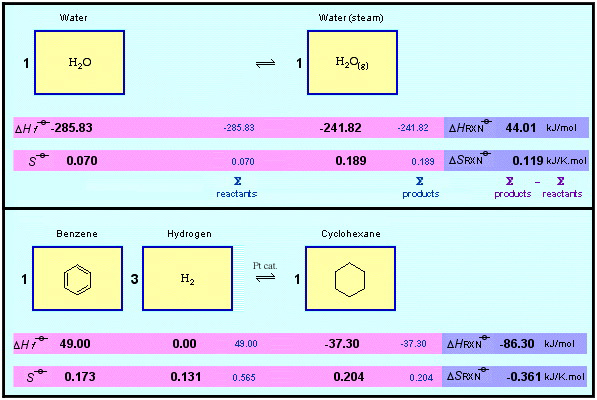
Seen in this light, evaporation and hydrogenation are both are phase change processes. Hence, it is very difficult – and not very useful – to distinguish between "physical" and "chemical" change. It is better to regard any phenomena that can be described in terms of a chemical equation as representing change in phase space.
Conclusions:
Linear chemical phenomena are commonly found in systems where the phase space is approaching, is close to, approximates to, or is at thermodynamic equilibrium.
Chemical equations are a concise way of showing changes in the phase space.
Electron and Proton Transfer
As discussed elsewhere in the chemogenesis web book, here, all reaction mechanisms can be broken down into STAD (substitution-transfer-abstraction-displacement) steps.
Special amongst all the various possible types of STAD step are electron, e–, transfer and proton, H+, transfer. This is because because in these two cases the transferring moiety is:
- very small
- low mass
- electronically simple
As a result e– and H+ transfers have few kinetic constraints (at least, at room temperature and in homogeneous solution) so a reaction's thermodynamic equilibrium position is rapidly reached. As a consequence, a huge amount of chemistry can be predicted using tabulated data and simple relationships.
Electron, e–, transfer equates with oxidation and reduction (redox) chemistry. Much redox chemistry can be predicted using the electrochemical series , a scale in which the standard hydrogen electrode is defined as having an E° of 0.00V. The associated Nernst equation models variations in concentration and temperature.
Proton, H+, transfer equates with Brønsted acid/base chemistry, here. Hydrogen ion concentration [H+] and pH are precisely defined measures of acidity and alkalinity. The pH of a wide variety of aqueous solutions, including buffer solutions, can be calculated from a knowledge of an acid's Ka or pKa. The logic extends to non-aqueous systems and is routinely employed by synthetic chemists who have a range of super acids and super bases at their disposal.
Electron and proton transfer reactions can be described in terms of the full equation, the net ionic equation and the half reactions. The half-reactions can then be arranged in a standard form:
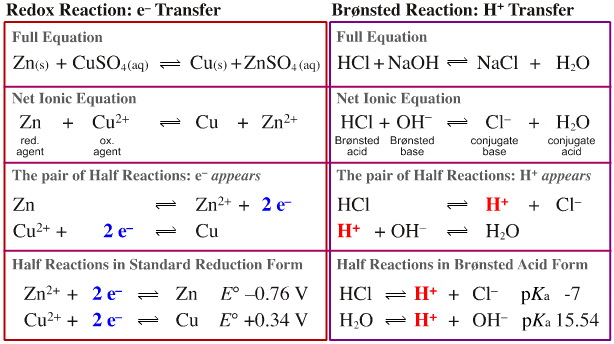
Conclusion:
Electron transfer and proton transfer processes are often predictable.
The Periodic Table
In the first half of twentieth century, much effort was expended trying to make the periodic table of the elements axiomatic, in other words, trying to fully understand the Mendeleev system in terms of a deeper theory, that deeper theory being quantum mechanics. Paul Dirac famously claimed this situation had been fully and completely achieved in principle:
"The underlying physical laws necessary for the mathematical theory of a large part of physics and the whole of chemistry are thus completely known and the difficulty is only that the exact application of these laws leads to equations much too complicated to be soluble." P.A.M. Dirac, Proc.R.Soc.Lond.Ser.A 123 (1929) 714
Dirac was obviously brilliant man – he devised the relativistic wave equation and predicted the existence of the positron – but he was not a complexity theorist.
We certainly teach our school and university students that the periodic table is explained in terms of electronic theory, and this line is advanced elsewhere in this web book, here,
The argument proffered is that: the pattern of spectral lines experientially obtained from a sample gas phase atoms (mono atomic) of an element, can be "explained by" (mapped to) quantum mechanics in the form of the Schrödinger wave equation, and the spectral lines and quantum patterns obtained by experiment and theory can be mapped to the Mendeleev Periodic Table of the Elements.
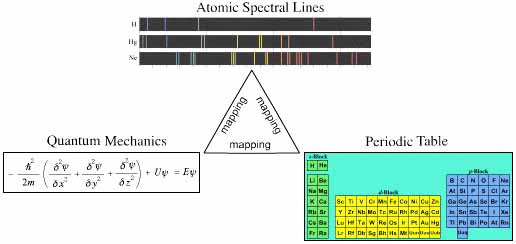
Much of this logic – in some detail – appears on the HyperPhysics site, here.
The relationship between electronic theory and atomic spectra is linear in the sense that there is a [close to] one-to-one mapping between theory and experiment, in exactly the same way that there is a one-to-one mapping between behaviour of a real gas in a piston and the ideal gas equation, PV = nRT. However, there is one essential difference: atoms, spectral lines and solutions to the wave equation (wavefunctions) are discrete quantised entities, whereas the classical behaviour of a gas in a piston is continuous.
Conclusion:
The periodic table of mono-atomic gas phase elements is axiomatic – linear with respect to theory – in that theory can predict behaviour and behaviour can be explained in terms of theory.
However...
Contrary to what we teach, Eric Scerri disputes that the full and complete axiomatic mapping between theory and the periodic table has been achieved. With good evidence he is able to say: "Electronic configurations are not [fully] reduced to quantum mechanics nor can they be derived from any other theoretical approach. They are obtained by a mixture of spectroscopic observations and semi-empirical methods like Bohr's aufbau scheme".Read the paper on-line: Eric R. Scerri, Has The Periodic Table Been Fully Axiomatized?, Erkenntnis, 47, 229-243, 1997.
One reason for the discrepancy between the full mapping we teach our students, and the incomplete mapping that worries Eric Scerri, this author and others – concerns multi-electron atoms, ions and molecules.
The point is that the Schrödinger wave equation can only be solved analytically for one electron systems like the hydrogen-atom, H•, and other one electron systems: He+, Li2+, Be3+, etc. For all multi-electron systems, approximations in the math have to be made to deal with electron/electron interactions and correlation. The mathematical techniques employed are usually pragmatic rather than rigorous, and they are often semi-empirical (partially based on experimental data). The effect is to produce nice fast computer code that efficiently predicts molecular energies, geometries and spectra, but at the expense of the theory being fully axiomatic: the logic becomes blurred.
Periodicity and Congeneric Series
Mendeleev's periodic law stated: "The properties of the elements are a periodic function of atomic mass."
This text has since been modified to: "The properties of the elements are a periodic function of atomic number" because atomic number (or proton number) is a more fundamental property than atomic mass.
Periodic trends can be addressed with a general diagram of the type:

Note that the periodic table can be formulated in various ways, and some more conducive to showing periodicity than others, hence the highly generalised version given above.
There is a nice page of periodicity links here.
Periodicity is usually discussed with reference to the chemical elements in the form of gas phase, mono atomic species, Na, Cl, etc., (this certainly the case for ionisation energy data), and sometimes as simple ions, Na+, Cl–, etc. In a few cases the periodic analysis extends to mono-elemental molecules, for example the physical and reaction chemistry of the diatomic halogens: F2, Cl2, Br2& I.
One of the aims of this Chemogenesis web book has been to extend the idea of periodicity. This has been achieved by employing a combinatorial analysis of the main group elements, normalised as the corresponding main group elemental hydrides, here. One of the benefits of this new analysis is that periodicity has been naturally extended into molecular and ionic space by identifying "congeneric" dots, series, planars & volumes of isoelectronic, isostructural & isoreactive species.
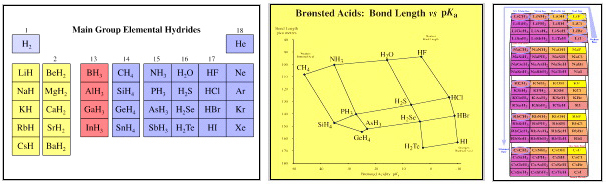
The Chemical Thesaurus Reaction Chemistry Database lists more than 100 congeneric series, planars and volumes.
Conclusion:
Periodic and congeneric structural and reactivity trends are linearities in reaction chemistry space.
These linearities are an echo of the patterns that exist in the electronic structure of atoms; they are fossils from an otherwise unseen quantum world.
Material Types: Elements & Binary Compounds
The chemical elements and binary elemental compounds present as real materials which may be: metallic, ionic, molecular covalent or network covalent, or intermediate between these four extreme types. Bonding and material type can be mapped to the van Arkel-Ketelaar triangle, here, and Laing tetrahedron, here:
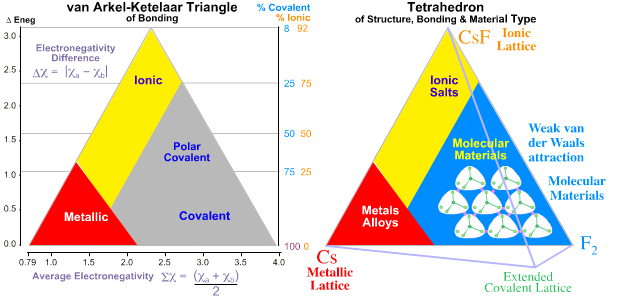
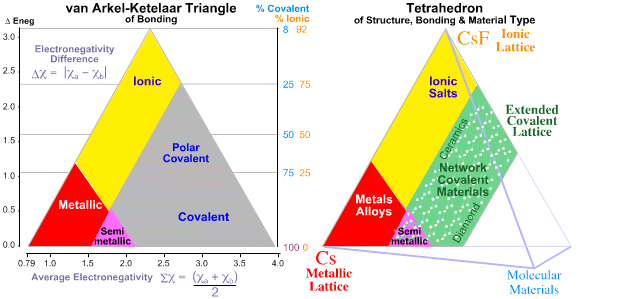
The bulk properties of elemental and binary materials can be "largely explained" in terms of electronegativity, van der Waals radii and ad hoc valency ruled bases on Lewis theory, and there is "good" – but NOT perfect – correlation between theory and material type.
Materials are commonly and rather successfully modelled in terms of molecular mechanics, a development of valence shell electron pair repulsion (VSEPR) theory.
Conclusion:
Metals, alloys, ionic materials, ceramics, polymers, bond polarity, molecular dynamics, crystal structure, etc., can all be "rather successfully" modelled using classical structural theory based on empirical evidence: atomic/ionic radii, bond lengths & angles, and electronegativity.
There is no need to resort to quantum mechanics, although this more sophisticated approach is available when the classical approach breaks down... which it invariably does.
Functional Groups, Homologous Series, Chemical Automata & Gliders
A computer based cellular automata use simple rules to turn squares on a screen on and off. Conway's Game of Life, above has two rules:
If a square is off, it turns on if exactly three of its neighbours are on. And, if a square is on, it stays on if exactly two or three neighbours are on, otherwise it turns off.
and four types of ending. An on screen object may:
- Grow for a few generations, then becomes static and unchanging.
- Becomes a "blinker".
- Grow for a few generations and then shrink, self-destruct and vanish.
- Eject a "glider", a Game of Life term, which moves away from the place where it formed until it collides with the edge of the playing area.
As a first approximation, atom–atom bonding is governed by two [sets of] rules:
• Metallic, ionic, covalent bond character is determined by (maps to) electronegativity
• Valency, in the form of VSEPR theory, determines covalent connectivity
Atomic interactions can be regarded as a type of cellular automata, and they too exhibit various common "end games".
- Consider a system with two hypothetical atoms, X and Y, which have electronegativity values of between 2.0 and 3.5 (This is so all bonding is unambiguously covalent.
- The atoms can have valances of 1, 2, 3 or 4, and all the various valency combinations occur.
- There are many exceptions and special cases, as discussed in more detail here, but commonly the interactions either give rise to either molecular materials or to network covalent materials:
|
1:1
|
XY
|
molecular
|
HCl
|
|
1:2
|
X2Y
|
molecular
|
H2O
|
|
1:3
|
X3Y
|
molecular
|
NH3
|
|
1:4
|
X4Y
|
molecular
|
CH4
|
|
2:2
|
XY
|
molecular
|
few
good examples: SO, O2
|
|
2:3
|
X3Y2
|
network
|
As2S3
|
|
2:4
|
X2Y
|
molecular
or network
|
CO2 or SiO2
|
|
3:3
|
XY
|
network
|
AsSb
|
|
3:4
|
X4Y3
|
network
|
Si3N4
|
|
4:4
|
XY
|
network
|
SiC
|
However, the analysis used on the Laing tetrahedron page, here, had the crucial constraint that compounds should only possess one type of chemical bond so that methane, CH4, was allowed, but that ethane, CH3CH3, and propane, CH3CH2CH3, which have both C–C and C–H bonds, were excluded.
Once the single-bond-type-constraint is removed the number of structural possibilities explodes! This is illustrated with the hydrocarbons, a set of molecular species containing only hydrogen and carbon, and where hydrogen has a valency of one and carbon has a valency of four.
The simplest hydrocarbon is methane, CH4. Next comes ethane, CH3CH3, then propane, CH3CH2CH3.
As the number of four valent carbon atoms increases, the connectivity rules allow for the existence of "isomers", which are compounds with the same molecular formula but with different structural formulae (a different connectivity). For example, C4H10 can exist as either butane, CH3CH2CH2CH3 or methylpropane (isobutane), CH3CH(CH3)CH3.
The number of possible structural isomers increases rapidly with molecular size:
|
Formula
|
Number
of Structural Isomers
|
|
CH4
|
1
|
|
C2H6
|
1
|
|
C3H8
|
1
|
|
C4H10
|
2
|
|
C5H12
|
3
|
|
C6H14
|
4
|
|
C7H16
|
5
|
|
C10H22
|
75
|
|
C20H42
|
366319
|
|
C30H62
|
>4
billion
|
Note that as molecular size increases some of the isomers will be structurally impossible for steric reasons.
Homologous series are "[sets] of compounds in which each member differs from the next by a constant amount" (Morrison & Boyd 5th Ed).
The "constant amount" is usually a –CH2– (methylene) function, and the simplest, archetypal, homologous series is observed with the set of linear alkanes:
|
methane
|
CH4
|
|
ethane
|
CH3CH3
|
|
propane
|
CH3CH2CH3
|
|
butane
|
CH3CH2CH2CH3
|
|
pentane
|
CH3CH2CH2CH2CH3
|
|
hexane
|
CH3CH2CH2CH2CH2CH3
|
|
heptane
|
CH3CH2CH2CH2CH2CH2CH3
|
Some fantastic patterns can emerge in this space. Consider the set of branched alkanes:
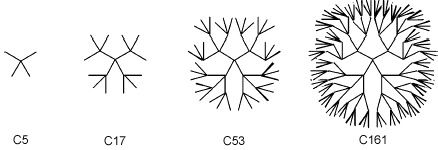
The structural formulae associated with the homologous series make a really rather cool pattern, so it is a great pity that only the C1 and C5 substances are known, here:
|
Structural Formula
|
Number of Carbons
|
|
CH4
|
1
|
|
C(CH3)4
|
5
|
|
C(C(CH3)3)4
|
17
|
|
C(C(C(CH3)3)3)4
|
53
|
|
C(C(C(C(CH3)3)3)3)4
|
161
|
|
C(C(C(C(C(CH3)3)3)3)3)4
|
485
|
|
C(C(C(C(C(C(CH3)3)3)3)3)3)4
|
1457
|
|
C(C(C(C(C(C(C(CH3)3)3)3)3)3)3)4
|
4373
|
|
C(C(C(C(C(C(C(C(CH3)3)3)3)3)3)3)3)4
|
13121
|
|
C(C(C(C(C(C(C(C(C(CH3)3)3)3)3)3)3)3)3)4
|
39365
|
Teachers, an interesting "lateral thinking" homework assignment. Get your students to deduce a formula to calculate the number of carbon atoms in the above homologous series. A spreadsheet giving the author's method is available on request.
One of the ideas central to organic chemistry is that of functional groups being "attached to" or "superimposed upon" a hydrocarbon sigma-skeleton. Examples include: alcohol, –OH, aldehyde, –CHO and carboxylic acid, –COOH, functions. When functional groups are appropriately attached to linear alkanes, new homologous series are produced:
methanol, ethanol, 1-propanol, 1-butanol, 1-pentanol, 1-hexanol...
methanal, ethanal, propanal, butanal, pentanal, hexanal...
methanoic acid, ethanoic acid, propanoic acid, butanoic acid, pentanoic acid, hexanoic acid...
Crucially, homologous series exhibit patterns of behaviour in terms of: boiling point, solubility, partition coefficient, reactivity, etc., which correlate with chain length and molecular weight.
Conclusion:
Homologous series are "gliders" (in the Game of Life sense) which project genuine linear structure and interaction behaviour into reaction chemistry space.
Scale Up
The ability to scale up reaction chemistry is extraordinary. If a chemical reaction takes in the lab using one gram amounts of material, we can say with great confidence that the reaction will also work out on the chemical plant (in the factory) on a multi tonne scale.
1.0 gram of cyclohexanol (GFW 100.2) contains 6 x 1021 molecules, and 1.0 tonne of cyclohexanol contains 6 x 1027 molecules, both are huge numbers and correspondingly the the entities exhibit very similar behaviours. Thus, the oxidation of cyclohexanol to cyclohexanone proceeds in a similar manner on both gram and tonne scales, a scale up factor of a million.
The counter example should make this point clear. The bridge designer cannot simply design a new bridge ten times bigger than the last one by multiplying all the dimensions by a factor of 10, let alone a million!
There are several provisos:
- Reagents which may seem moderately priced in the lab, may not be economically viable on a large scale.
- Exothermic reactions may need to be cooled, and/or the rate of addition of the reagents need to be carefully monitored and controlled. With exothermic reactions there is always the risk of reaction "runaway" leading to explosion.
- There will be health and safety issues.
- Waste must be safely disposed of.
- There are a number of common lab techniques and pieces of equipment do not scale well:
- Filtration can be a problem at any scale, and on a bigger scale it will be a bigger problem.
- There is no ton scale equivalent of the rotary evaporator, one of the most useful bits of kit in the synthetic chemistry lab.
- Chromatography is an excellent analytical technique, but industrial scale preparative chromatography always gives problems.
Nuclear Chemistry Scale UpIn his 1986 Pulitzer prize winning book The Making of The Atomic Bomb, Richard Rhodes tells the story of how plutonium, Pu-239, became the preferred nuclear explosive over uranium 235. Uranium is mined, but plutonium is formed in a nuclear reactor. However, it is easier to chemically extract Pu from the zoo of other elements present by selective precipitation and extraction techniques, than it is than to physically separate the U-235 from U-238 by physical means. In other words, the chemistry scales better than the physics!
|
The reason why chemical reactions scale so well is that they are inherently parallel processes in which billions upon billions of independent chemical species interact and react [more or less] simultaneously.
Conversely, most engineering processes are serial, with production one-at-a-time at a time on an assembly line. Houses, bridges, cars, computers are made in a serial way.
A notable exception is seen with the manufacture of the silicon microprocessor chips that power so much modern technology. Here hundreds of microprocessor, memory & logic components are made in parallel on large silicon wafers, which may be up to 300mm in diameter.
This author sees the biggest issue facing nanotechnology concerns scale-up. Go to the abstract page of the journal NanoLetters and take a look at recent advances. Ask yourself how well the experiments discussed will scale. I suggest some will scale well, but many won't.
Conclusion:
In principle and practice, chemical reaction processes can be scaled up from atom + atom to multi tonne.
Quantitative Structure Activity Relationships (QSAR)
"A QSAR [project] attempts to find consistent [linear] relationships between the variations in the values of molecular properties and the biological activity for a series of compounds, so that these rules can be used to evaluate new chemical entities". From an excellent Introduction to QSAR Methodology on the Network Science site.
Finding a linear relationship between molecular structure and pharmaceutical activity is the Holy Grail of drug discovery research.
Conclusion:
Linearities exist in pharmacology space in terms of dose-response and structure-activity, but these are outside the scope of this webbook.
Molecular Biology
Molecular biology appears to use chemical interaction in a very "digital" way. DNA replication & repair, protein synthesis, hormone/receptor & protein/protein interactions, the polymerase chain reaction, the passing of nerve signals across synaptic junctions, etc., etc., etc., all involve molecular recognition. The processes seem to proceed by a kind of molecular-clockwork designed and maintained by a Blind Watchmaker.
Briefly: DNA codes for mRNA, then at the ribosome mRNA codes for the sequence of amino acids which make up the peptide chain and primary protein structure. There is a formal correspondence between the four letter language of the generic code the 20 letter code leading to amino acid sequence and protein structure.
These processes seems to have more in common with a computer program than compressing a sample of gas in a piston to check the validity of the ideal gas equation, PV = nRT.
It seems [and here the author is writing as a spectator, not a participant] that biological systems use "analogue" mechanisms, such as sensing and responding to concentration gradients, in ways that are of secondary importance compared with "digital" DNA replication and protein synthesis.
Biological chemistry, and even biology, can be very reproducible, linear and predictable: Yeast cells replicate to (what appear to be) identical yeast cells, and yeast cells ferment aqueous sugar solutions to ethanol with high reproducibility.
Conclusion:
The linearities are associated with the pattern recognition logic of molecular biology. These linearities are emergent.
Linear Chemistry: Summing Up
The linear regions of behaviour in chemistry space are so incredibility important to science, technology/engineering and education that practitioners tend to concentrate on the linear regions. The effect is that chemistry space appears to be more linear and predictable than it actually is.
- Scientists
and philosophers of science are interested in linear behaviour because
linear data can be converted to models, theories and laws, and this
profound knowledge is used to help explain the world we inhabit.
- Engineers
and technologists are always keen to exploit regions where behaviour
is stable and predictable. Bridges are seldom designed to be on point
of collapse, and likewise, chemical engineers develop processes which
maximise yield and minimise the risk of explosion.
- Teachers concentrate on linear regions of chemical science because of their theoretical, practical and educational importance.
 |
 |
 |
| Chemistry & Complexity: Systems Thinking | Chemistry & Complexity: Non-Linear Systems |
© Mark R. Leach 1999 –
Queries, Suggestions, Bugs, Errors, Typos...
If you have any:
Queries
Comments
Suggestions
Suggestions for links
Bug, typo or grammatical error reports about this page,please contact Mark R. Leach, the author, using mark@meta-synthesis.com
This free, open access web book is an ongoing project and your input is appreciated.