Periodic Table |
 |
 |
 |
 |
 |
 |
 |
| Binary Compound Synthlet | van Arkel-Ketelaar Triangles |
Electronegativity
After atomic number, mass & valency, electronegativity is the most important of all atomic parameters. This page is expanded into a full paper, Electronegativity as a Basic Elemental Property, by Mark Leach available here (PDF).
The History of Electronegativity
It is a common misapprehension that Linus Pauling 'invented' electronegativity in 1932, but the idea pre-dates this time. In 1895 the Danish thermochemist Hans Peter Jørgen Julius Thomsen proposed a periodic table that shows electropositive and electronegative elements:
However, the concept of electronegativity was put on a quantitative footing in 1932 by Linus Pauling in The Nature of the Chemical Bond. IV. The Energy of Single Bonds and the Relative Electronegativity of Atoms, Journal of the American Chemical Society, 54, p. 3570-3582. The original paper is available in HTML here.
In his textbook The Nature of The Chemical Bond (published 1938, quote from 3rd ed.), Pauling says about electronegativity:
"The power of an atom in a molecule to attract electrons to itself."
In his General Chemistry textbook (pp 183) Pauling writes:
"It has been found possible to assign to the elements numbers representing their power of attraction for the electrons in a covalent bond, by means of which the amount of partial ionic character may be estimated."
The IUPAC Gold Book says:
"Concept introduced by L. Pauling as the power of an atom to attract electrons to itself. There are several definitions. According to Mulliken it is the average of the ionization energy and electron affinity of an atom, but more frequently a relative scale due to Pauling is used where dimensionless relative electronegativity differences are defined on the basis of bond dissociation energies", here.
As discussed here, this author's definition is:
"Electronegativity is a measure, integrated over numerous physical parameters, of the power of a gas phase or bonded atom to attract electrons to itself."
While not too much should be read into absolute values, many trends in structure and reactivity behaviour can be mapped to ("explained in terms of", or correlated with) Pauling's electronegativity data. This makes electronegativity an extraordinarily useful concept.
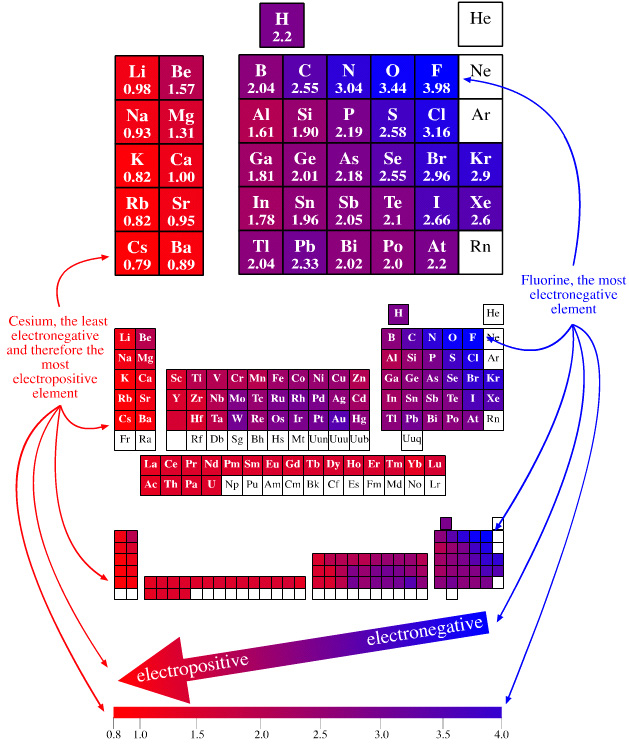
- There is a broad sweep of electronegativity from top-right to bottom-left. (The radioactive elements francium, Fr, & radium Ra, are ignored as are the lighter and unreactive Group 18 elements: helium, He, neon, Ne, and argon, Ar.)
- The electronegative elements, found top-right, present as non-metals. Fluorine, F2, oxygen, O2, & chlorine, Cl2, are strong oxidising agents: they accept electrons and are easily reduced. The electronegative elements all form negatively charged anions and they may form entities that interact via lone-pairs of electrons. Anions and electron lone pairs are associated with Lewis base behaviour.
- The electropositive elements all present as metals. Metals behave as electron donating reducing agents. Metals form cations that behave as Lewis acids.
- Hydrogen is shown above and between boron and carbon. This is because the C–H bond is polarised δ-C–Hδ+ and the B–H bond is polarised δ+B–Hδ–. IUPAC are considering the position of hydrogen on their official periodic table, here.
In this web book, electropositive elements are coloured
RED and electronegative elements are coloured BLUE. The
rational is that:
Using this colour representation, the top-right to bottom-left diagonal trend can be clearly seen across the main group elements and across the entire periodic table. |
Why Is Electronegativity Important?
The metallic elements are all electropositive, the electronegative elements are all non-metals, the metalloids are found at intermediate electronegativities.
Ionic compounds, like sodium chloride NaCl, or Na+ Cl–, are formed between between electropositive elements (Na, 0.93) and electronegative elements (Cl, 3.16).
Indeed, bond type, material character and chemical reactivity can be predicted from a knowledge of electronegativity.
For example, consider:
- Hydrogen chloride, HCl. Chlorine, 3.16, is more electronegative than hydrogen, 2.20, so the H–Cl bond will be polarised Hδ+–Clδ–. (This is pronounced "delta plus" and "delta minus".) This tells us that HCl will react as H+ and Cl–, and HCl is a proton donating Brønsted acid.
- Methyl bromide, CH3Br, has a C–Br bond that is polarised Cδ+–Brδ– and the carbon atom in the molecule is susceptible to nucleophilic substitution.
- There are many, many examples like this in main group and organic chemistry.
Where Do The Numbers Come From?
Pauling's empirical electronegativity scale is derived from thermochemical bond-energy data. Pauling observed that bond enthalpy, EA-B, in kcal/mol between atoms A and B can be predicted using the equation below, where ΧA and ΧB are the electronegativity values of A and B.
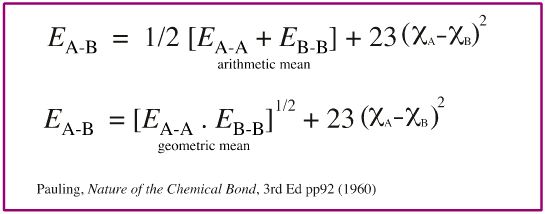
In his book The Nature of The Chemical Bond, Pauling comments that it is more accurate to use the geometric mean rather than the arithmetic mean, but then uses the arithmetic mean himself. Other authors note this and then also use the arithmetic mean.
Calculations for the formation of the halogen halides: HF, HCl, HBr & HI from hydrogen, H2, and the halogens, F2, Cl2, Br2& I2 show how the Pauling relationship compares with experimental data:
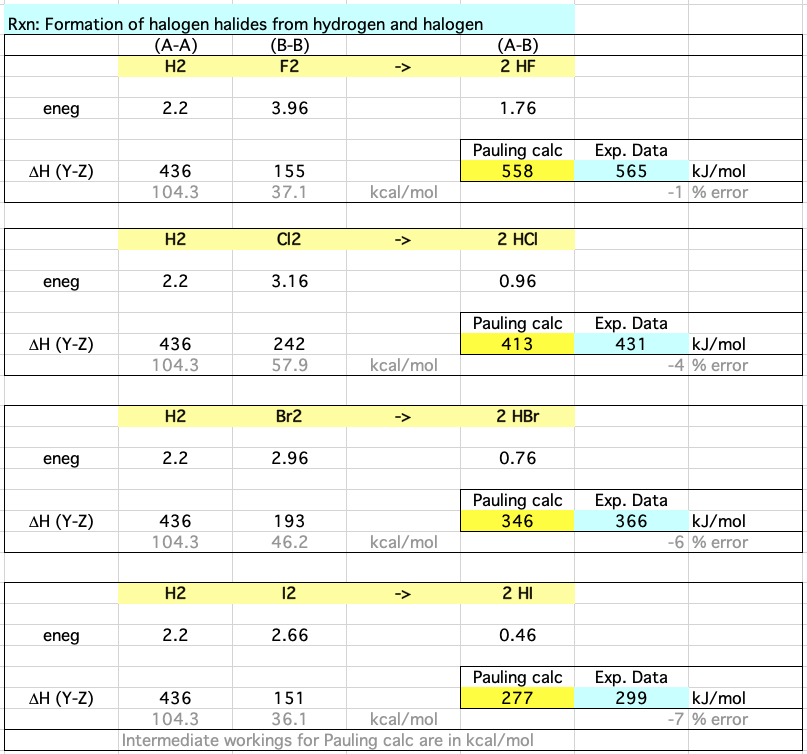
Download the Excel spreadsheet here. Data is from Pauling's Nature of the Chemical Bond. Note that the equation requires data to be in kcal/mol rather than kJ/mol.
The electronegativity difference between elements A and B is determined from the following relationships:

Note that (a) both the geometric and arithmetic mean relationships are given and (b) or many metals the enthalpy of salt formation data is used as a proxy.
Once a set of electronegativity differences are known, it is a simple matter to assign absolute electronegativity values.
Compounds & Materials, Structure & Reactivity
Chlorine, by way of example, is the third most electronegative element after fluorine and oxygen. This electronegative nature is apparent in the structure and reaction chemistry of:
- The small atomic radius of the chlorine atom, Cl•
- The dichlorine molecule, Cl2
- Ionic sodium chloride, NaCl
- Molecular chloromethane, CH3Cl
- etc.
Electronegativity can be used to predict the dipole moment (bond polarity) of a bond:
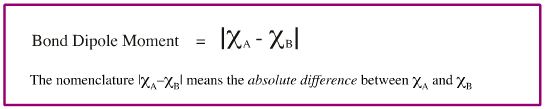
Electronegativity can be used to approximately predict the degree of ionic (and therefore covalent) character of a bond between two dissimilar elements:

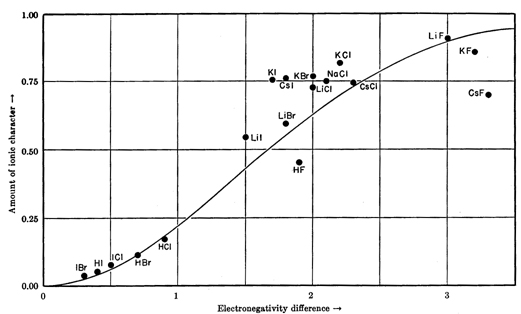
- Electronegativity can be
used to predict metallic, ionic, covalent and intermediate bond type,
and these behaviours can be mapped to the Van Arkel-Ketelaar Triangle
of Bonding, as discussed in detail on the next page of the Chemogenesis
web book, here.
- When valency is included
as an additional parameter, electronegativity can be mapped to the Tetrahedron of Structure, Bonding & Material Type, as discussed here.
- Electronegativity can be
used to predict chemical reactivity because:
"The most stable arrangement of [polar] covalent bonds connecting a group of atoms is that arrangement in which the atom with the highest electronegativity be bonded to the atom with the lowest electronegativity." Jolly, Modern Inorganic Chemistry, McGraw-Hill (1985) pp 61-62.
It follows that pairs of compounds of the type A-Bm and X-Yn will react with each other to maximise and minimise electronegativity difference, as discussed on this page of The Chemogenesis web book: Why Do Chemical Reactions Happen?, here.
- Electronegativity, along with bond-length, pKa and other data, is central to the chemogenesis analysis, as discussed in the sections of this web book: Quantifying Congeneric Behaviour and Congeneric Array Interactions, here and here.
Captured from The Nature of The Chemical Bond, 3rd Ed, pp99. The experimental values are from vapour phase dipole moments.
Electronegativity and Theory
Pauling used bond enthalpy data to construct his electronegativity scale. Other workers have used other starting points.
|
Eneg Scale
|
Method
|
| Pauling
Scale 1932 |
Obtains values by thermochemical methods. Paper |
| Mulliken
Relation 1934 |
Defines a relation that depends upon the orbital characteristics of an atom in a molecule. Mulliken electronegativity is the numerical average of the ionisation potential and electron affinity. Wikipedia |
| Gordy
Scale 1946 |
Defines
electronegativity in terms of the effective nuclear charge and the
covalent radius. (Zeff)e/r. Phys.
Rev. 69, 604 - 607 (1946) Gordy developed several scales! |
| Walsh
Scale 1951 |
Relates electronegativity to stretching force constants of the bonds of an atom to a hydrogen atom. Paper |
| Huggins
Scale 1953 |
Alternative to Pauling's thermochemical procedure. Discussed in this paper |
| Sanderson
Scale 1955 |
The ratio of the average electron density of an atom to that of a hypothetical "inert" atom having the same number of electrons. This ratio is a measure of the relative compactness of the atom. J.Chem.Phys. 23, 2467 (1955) |
| Allred-Rochow
Scale 1958 |
Defines electronegativity in terms of the effective nuclear charge and covalent radius. Like the Gordy scale but uses (Zeff)e/r2. Wikipedia |
| Jaffe
Scale 1962 |
Uses the electronegativity of orbitals rather than atoms to develop group electronegativities for molecular fragments (eg. CH3 vs CF3) that take into account the charge of a group, the effects of substituents, and the hybridization of the bonding orbital. Electronegativity. I. Orbital Electronegativity of Neutral Atoms J. Hinze and H.H.Jaffe, J.Am.Chem. Soc., 1962, 84, 540 |
| Phillips
Scale 1968 |
Defines electronegativity in terms of the dielectric properties of atoms in a given valence state. Paper |
| Martynov
& Batsanov Scale 1980 |
Obtained by averaging the successive ionisation energies of an element's valence electrons. Russ. J. Inorg. Chem., 1980, 25, 1737. |
| Allen
CE Scale 1992 |
Configuration energy (CE), the average one-electron valence shell energy of the ground-state free atom, Electronegativity is the average one-electron energy of the valence-shell electrons in ground-state free atoms, Leland C. Allen, J. Am. Chem. Soc. 1989, 111, 25, 9003-9014, is used to quantify metal-covalent-ionic bonding, Extension and completion of the periodic table, Leland C. Allen, J.Am.Chem.Soc., (1992), 114, 1510 |
Lang & Smith |
Peter F. Lang & Barry C. Smith presented a paper: An equation to calculate internuclear distances of covalent, ionic and metallic lattices, Phys. Chem. Chem. Phys., 2015, 17, 3355. Quoted from the paper:
|
More in: H.B. Michaelson, IBM J. Res. Develop. 22 1 (1978). Review article by H. O. Pritchard and H. A. Skinner: The Concept Of Electronegativity, Chem. Rev.; 1955; 55(4) pp 745 - 786.
Electronegativity seems to integrate (average out) a number of arcane atomic electronic parameters. It is a proxy parameter that in a rather simple way maps to chemical structure and reactivity.
In his 1992 paper (J.Am.Chem.Soc., (1992), 114, 1510), Allen argued that configuration energy, CE, is a fundamental atomic property and is the "missing third dimension to the periodic table". He further stated that electronegativity is an 'ad hoc' parameter. More usefully – in this author's judgment– Allen's work shows that configuration energy, CE, correlates with electronegativity.
Indeed, electronegativity is so important that in this author's judgment it should be considered to be a basic atomic property rather than a simple atomic property, here.
In 1960 Pauling defined electronegativity as:
"The power of an atom in a molecule to attract electrons to itself"
However, when considered in the context of semiquantitative tetrahedra of structure of bonding and material type, this statement is literally too narrow because bulk binary compounds can be metallic, ionic or network covalent as well as molecular.
Any definition of electronegativity must not be self-limiting. An updated definition [propsed here] is:
"Electronegativity is a measure, integrated over numerous physical parameters, of the power of a gas phase or bonded atom to attract electrons to itself."
Tables of Electronegativity Data
Electronegativity |
Pauling |
Revised Pauling |
Sanderson |
Lang & Smith |
||||
1 |
H |
Hydrogen | 2.1 |
2.20 |
2.8 |
2.31 |
2.20 |
2.00 |
2 |
He |
Helium | ||||||
3 |
Li |
Lithium | 1.0 |
0.98 |
1.3 |
0.86 |
0.97 |
1.24 |
4 |
Be |
Beryllium | 1.5 |
1.57 |
1.61 |
1.47 |
2.14 |
|
5 |
B |
Boron | 2.0 |
2.04 |
1.8 |
1.88 |
2.01 |
1.81 |
6 |
C |
Carbon | 2.5 |
2.55 |
2.5 |
2.47 |
2.50 |
2.30 |
7 |
N |
Nitrogen | 3.0 |
3.04 |
2.9 |
2.93 |
3.07 |
2.82 |
8 |
O |
Oxygen | 3.5 |
3.44 |
3.0 |
3.46 |
3.50 |
3.39 |
9 |
F |
Fluorine | 4.0 |
3.98 |
4.1 |
3.92 |
4.10 |
4.00 |
10 |
Ne |
Neon | ||||||
11 |
Na |
Sodium | 0.9 |
0.93 |
1.2 |
0.85 |
1.01 |
1.18 |
12 |
Mg |
Magnesium | 1.2 |
1.31 |
1.42 |
1.23 |
1.76 |
|
13 |
Al |
Aluminum | 1.5 |
1.61 |
1.4 |
1.54 |
1.47 |
1.31 |
14 |
Si |
Silicon | 1.8 |
1.90 |
2.0 |
1.74 |
1.74 |
1.66 |
15 |
P |
Phosphorus | 2.1 |
2.19 |
2.3 |
2.16 |
2.06 |
2.05 |
16 |
S |
Sulfur | 2.5 |
2.58 |
2.5 |
2.66 |
2.44 |
2.49 |
17 |
Cl |
Chlorine | 3.0 |
3.16 |
3.3 |
3.28 |
2.83 |
2.95 |
18 |
Ar |
Argon | 3.92 |
|||||
19 |
K |
Potassium | 0.8 |
0.82 |
1.1 |
0.74 |
0.91 |
1.00 |
20 |
Ca |
Calcium | 1.0 |
1.00 |
1.06 |
1.04 |
1.40 |
|
21 |
Sc |
Scandium | 1.3 |
1.36 |
1.09 |
1.20 |
1.51 |
|
22 |
Ti |
Titanium | 1.5 |
1.54 |
1.57 | |||
23 |
V |
Vanadium | 1.6 |
1.63 |
1.62 | |||
24 |
Cr |
Chromium | 1.6 |
1.66 |
1.65 | |||
25 |
Mn |
Manganese | 1.5 |
1.55 |
1.71 | |||
26 |
Fe |
Iron | 1.8 |
1.83 |
1.77 | |||
27 |
Co |
Cobalt | 1.8 |
1.88 |
1.84 | |||
28 |
Ni |
Nickel | 1.8 |
1.91 |
1.92 | |||
29 |
Cu |
Copper | 1.9 |
1.90 |
2.02 | |||
30 |
Zn |
Zinc | 1.6 |
1.65 |
1.86 |
1.66 |
2.16 |
|
31 |
Ga |
Gallium | 1.6 |
1.81 |
1.4 |
2.10 |
1.82 |
1.31 |
32 |
Ge |
Germanium | 1.8 |
2.01 |
1.9 |
2.31 |
2.02 |
1.62 |
33 |
As |
Arsenic | 2.0 |
2.18 |
2.2 |
2.53 |
2.20 |
1.95 |
34 |
Se |
Selenium | 2.4 |
2.55 |
2.4 |
2.76 |
2.48 |
2.30 |
35 |
Br |
Bromine | 2.8 |
2.96 |
3.0 |
2.96 |
2.74 |
2.67 |
36 |
Kr |
Krypton | 2.90 |
3.17 |
||||
37 |
Rb |
Rubidium | 0.8 |
0.82 |
1.0 |
0.70 |
0.89 |
0.96 |
38 |
Sr |
Strontium | 1.0 |
0.95 |
0.96 |
0.99 |
1.31 |
|
39 |
Y |
Yttrium | 1.2 |
1.22 |
1.4 |
0.98 |
1.11 |
1.54 |
40 |
Zr |
Zirconium | 1.4 |
1.33 |
1.57 | |||
41 |
Nb |
Niobium | 1.6 |
1.60 |
1.61 | |||
42 |
Mo |
Molybdenum | 1.8 |
2.16 |
1.66 | |||
43 |
Tc |
Technetium | 1.9 |
1.90 |
1.71 | |||
44 |
Ru |
Ruthenium | 2.2 |
2.20 |
1.76 | |||
45 |
Rh |
Rhodium | 2.2 |
2.28 |
1.84 | |||
46 |
Pd |
Palladium | 2.2 |
2.20 |
1.91 | |||
47 |
Ag |
Silver | 1.9 |
1.93 |
1.92 | |||
48 |
Cd |
Cadmium | 1.7 |
1.69 |
1.73 |
1.46 |
2.06 |
|
49 |
In |
Indium | 1.7 |
1.78 |
1.3 |
1.88 |
1.49 |
1.26 |
50 |
Sn |
Tin | 1.8 |
1.96 |
1.8 |
2.02 |
1.72 |
1.49 |
51 |
Sb |
Antimony | 1.9 |
2.05 |
2.0 |
2.19 |
1.82 |
1.73 |
52 |
Te |
Tellurium | 2.1 |
2.10 |
2.2 |
2.34 |
2.01 |
2.01 |
53 |
I |
Iodine | 2.5 |
2.66 |
2.7 |
2.50 |
2.21 |
2.32 |
54 |
Xe |
Xenon | 2.63 |
|||||
55 |
Cs |
Cesium | 0.7 |
0.79 |
1.0 |
0.69 |
0.86 |
0.89 |
56 |
Ba |
Barium | 0.9 |
0.89 |
0.93 |
0.97 |
1.20 |
|
57 |
La |
Lanthanum | 1.1 |
1.10 |
0.92 |
1.08 |
1.28 |
|
58 |
Ce |
Cerium | 1.1 |
1.12 |
1.27 | |||
59 |
Pr |
Praseodymium | 1.1 |
1.13 |
1.25 | |||
60 |
Nd |
Neodymium | 1.1 |
1.14 |
1.27 | |||
61 |
Pm |
Promethium | 1.1 |
1.13 |
1.28 | |||
62 |
Sm |
Samarium | 1.1 |
1.17 |
1.30 | |||
63 |
Eu |
Europium | 1.1 |
1.20 |
1.30 | |||
64 |
Gd |
Gadolinium | 1.1 |
1.20 |
1.41 | |||
65 |
Tb |
Terbium | 1.1 |
1.20 |
1.35 | |||
66 |
Dy |
Dysprosium | 1.1 |
1.22 |
1.36 | |||
67 |
Ho |
Holmium | 1.1 |
1.23 |
1.38 | |||
68 |
Er |
Erbium | 1.1 |
1.24 |
1.40 | |||
69 |
Tm |
Thulium | 1.1 |
1.25 |
1.42 | |||
70 |
Yb |
Ytterbium | 1.1 |
1.10 |
1.44 | |||
71 |
Lu |
Lutetium | 1.1 |
1.27 |
1.25 | |||
72 |
Hf |
Hafnium | 1.3 |
1.30 |
1.57 | |||
73 |
Ta |
Tantalum | 1.5 |
1.50 |
1.73 | |||
74 |
W |
Tungsten | 1.7 |
2.36 |
1.81 | |||
75 |
Re |
Rhenium | 1.9 |
1.90 |
1.80 | |||
76 |
Os |
Osmium | 2.2 |
2.20 |
1.94 | |||
77 |
Ir |
Iridium | 2.2 |
2.20 |
2.06 | |||
78 |
Pt |
Platinum | 2.2 |
2.28 |
2.06 | |||
79 |
Au |
Gold | 2.4 |
2.54 |
2.12 | |||
80 |
Hg |
Mercury | 1.9 |
2.00 |
1.92 |
1.44 |
2.40 |
|
81 |
Tl |
Thallium | 1.8 |
2.04 |
1.96 |
1.44 |
1.34 |
|
82 |
Pb |
Lead | 1.8 |
2.33 |
2.01 |
1.55 |
1.51 |
|
83 |
Bi |
Bismuth | 1.9 |
2.02 |
2.06 |
1.67 |
1.68 |
|
84 |
Po |
Polonium | 2.0 |
2.00 |
1.90 | |||
85 |
At |
Astatine | 2.2 |
2.20 |
2.12 | |||
86 |
Rn |
Radon | ||||||
87 |
Fr |
Francium | 0.7 |
0.70 |
||||
88 |
Ra |
Radium | 0.9 |
0.90 |
||||
89 |
Ac |
Actinium | 1.1 |
1.10 |
||||
90 |
Th |
Thorium | 1.3 |
1.30 |
||||
91 |
Pa |
Protactinium | 1.5 |
1.50 |
||||
92 |
U |
Uranium | 1.7 |
1.38 |
||||
93 |
Np |
Neptunium | 1.3 |
1.36 |
||||
94 |
Pu |
Plutonium | 1.3 |
1.28 |
||||
A plot of the above data shows that, broadly, the various electronegativity systems are numerically equivalent:
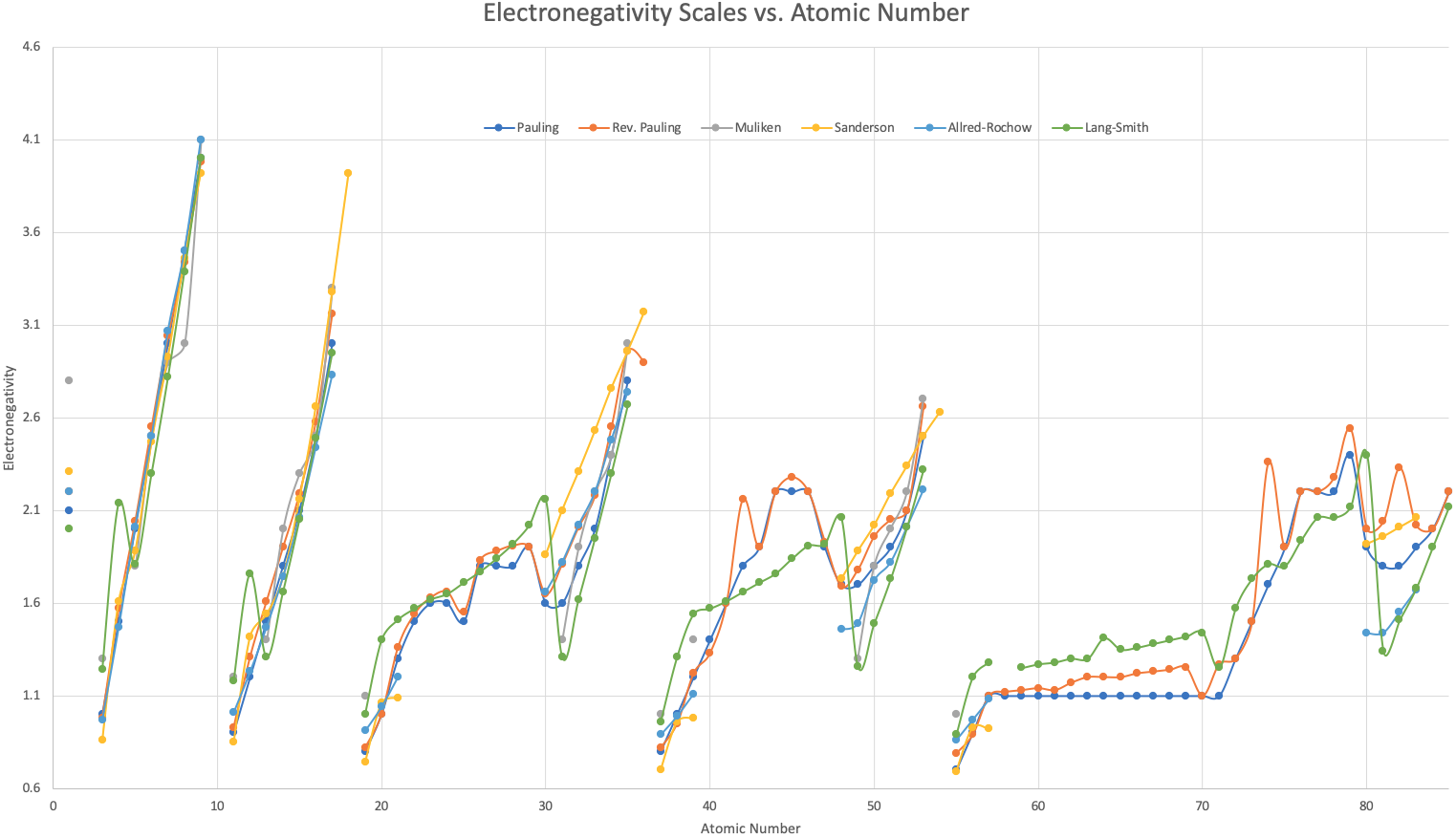
This page is expanded into a full paper, Electronegativity as a Basic Elemental Property, by Mark Leach available here.
Some recommended Wikipedia links:
Download an electronegativity & bond character calculator spreadsheet, here.
Many thanks to Bruce Railsback for his helpful comments about this page.
 |
 |
 |
| Binary Compound Synthlet | van Arkel-Ketelaar Triangles |
© Mark R. Leach 1999 –
Queries, Suggestions, Bugs, Errors, Typos...
If you have any:
Queries
Comments
Suggestions
Suggestions for links
Bug, typo or grammatical error reports about this page,please contact Mark R. Leach, the author, using mark@meta-synthesis.com
This free, open access web book is an ongoing project and your input is appreciated.