• meta-synthesis
• About
  About meta-synthesis Reviews Products & Services Contact Us FAQs
• Our Websites
  The INTERNET Database of Periodic Tables The Chemogenesis Web Book The Chemical Thesaurus Chemistry Tutorials & Drills The Truncated Tetrahedron Orange Gate Journal George Truefitt FRIBA Mac Ruff's PNG Sketchbooks MRL's Bow Tuning Page
• Web Book Chapters
  Web Book Home Page | Chemogenesis Main Index Foreword:  Chemogenesis & Chemical Education Part I   Atoms | Elements | Periodic Tables 1.1  Introduction to Chemogenesis 1.2  Nucleosynthesis of The Elements 1.3  The Segrè Chart 1.4  Quantum Numbers to Periodic Tables 1.5  The Periodic Table: What Is It Showing? 1.6  The INTERNET Database of Periodic Tables 1.7  Periodicity Part II   Structure | Bonding | Material Type 2.1  Mono-Bond Typed Binary Compounds 2.2  Binary Compound Synthlet 2.3  Electronegativity 2.4  van Arkel-Ketelaar Triangles of Bonding 2.5  Tetrahedra of Structure, Bonding & Material Type 2.6  Structure, Bonding & Material Synthlet 2.7  The Classification of Matter: Chemical Stuff Part III   Interactions | Reactions | Mechanisms 3.1  The Chemogenesis Analysis: An Overview 3.2  Lewis and Brønsted Models of Acidity 3.3  The Hard Soft [Lewis] Acid Base Principle 3.4  Main Group Elemental Hydrides 3.5  Five Hydrogen Probe Experiments 3.6  Congeneric Dots, Series & Planars 3.7  Quantifying Congeneric Behaviour 3.8  Ligand Replacement Congeneric Series 3.9  Congeneric Array Interactions 3.10  Emergence of Organic Chemistry 3.11  Congeneric Array Database 3.12  The Five Reaction Chemistries 3.13  Lewis Acids & Lewis Bases: A New Analysis 3.14  The Lewis Acid/Base Interaction Matrix 3.15  Patterns in Reaction Chemistry Poster 3.16  Lewis Acid/Base Interaction Matrix Database 3.17  Nucleophiles, Bases & The Fluoride Ion 3.18  Redox Chemistry 3.19  Redox Chemistry Synthlet 3.20  Radical Chemistry 3.21  Diradical Chemistry 3.22  Photochemistry 3.23  Species/Species Interactions 3.24  The Simplest Mechanistic Step: STAD 3.25  Unit & Compound Mechanistic Steps 3.26  The Mechanism Matrix 3.27  Addition To A Double Bond Synthlet 3.28  Pericyclic Reaction Chemistry Part IV   Chemical Theory 4.1  Why Do Chemical Reactions Occur? 4.2a  Thermochemistry: List Reactions Synthlet 4.2b  Thermochemistry: Play With Reaction Synthlet 4.2c  Thermochemistry: Bulid A Reaction Synthlet 4.3  Timeline of Structural Theory 4.4  Modern Lewis Theory 4.5  Valence Shell Electron Pair Repulsion: VSEPR 4.6  Diatomic Species by Molecular Orbital Theory 4.7  Polyatomic Species: Molecular Orbitals 4.8  Organic π-Systems 4.9  Functional Group Database Part V   Complexity & Emergence 5.1  Chemistry & Complexity: Systems 5.2  Linear Chemistry Systems 5.3  Chemical Systems Prone to Complexity Part VI   Extras 6.1  Chemogenesis Videos 6.2  Chemical Thesaurus Reaction Chemistry Database 6.3  Chemogenesis Paper 6.4  Electronegativity as a Basic Elemental Property 6.5  What is Chemistry? (Workshop) 6.6  Literature References & Further Reading

Periodic Table T-Shirts & more from the meta-synthesis   See More   Merch Store

The INTERNET Database of Periodic Tables

There are thousands of periodic tables in web space, but this is the only comprehensive database of periodic tables & periodic system formulations. If you know of an interesting periodic table that is missing, please contact the database curator: Mark R. Leach Ph.D.

Use the drop menus below to search & select from the more than 1300 Period Tables in the database: 

• SEARCH:
• By Decade
  Formulations 2020 to present day Formulations 2010 – 2019 Formulations 2000 – 2009 Formulations 1950 – 1999 Formulations 1900 – 1949 Formulations 1850 – 1899 Formulations 1800 – 1849 Formulations Before 1800
• By Type
  Periodic Tables showing Data Spiral & Helical Formulations 3-Dimensional Formulations Miscellaneous Periodic Tables Books & Reviews Non-Chemistry Periodic tables Top 10 Periodic Tables Recently Added Periodic Tables
• Pre-Selected
  Best Four Periodic Tables for Data All Periodic Tables by Name All Periodic Tables by Date All Periodic Tables by Reverse Date All Periodic Tables, as Added to the Database All Periodic Tables, reverse as Added Elements by Name Elements by Date Discovered Search for: Mendeleev/Mendeléeff Search for: Janet/Left-Step Search for: Eric Scerri Search for: Mark Leach Search for: René Vernon Search for: Electronegativity
• By Year
  2020 2019 2018 2017 2016 2015 2014 2013 2012 2011 2010 2009 2008 2007 2006 2005 2004 2003 2002 2001 2000 1999 1998 1997 1996 1995 1994 1993 1992 1991 1990 1989 1988 1987 1986 1985 1984 1983 1982 1981 1980 1979 1978 1977 1976 1975 1974 1973 1972 1971 1970 1969 1968 1967 1966 1965 1964 1963 1962 1961 1960 1959 1958 1957 1956 1955 1954 1953 1952 1951 1950 1949 1948 1947 1946 1945 1944 1943 1942 1941 1940 1939 1938 1937 1936 1935 1934 1933 1932 1931 1930 1929 1928 1927 1926 1925 1924 1923 1922 1921 1920 1919 1918 1917 1916 1915 1914 1913 1912 1911 1910 1909 1908 1907 1906 1905 1904 1903 1902 1901 1900 1899 1898 1897 1896 1895 1894 1893 1892 1891 1890 1889 1888 1887 1886 1885 1884 1883 1882 1881 1880 1879 1878 1877 1876 1875 1874 1873 1872 1871 1870 1869 1868 1867 1866 1865 1864 1863 1862 1861 1860 1859 1858 1857 1856 1855 1854 1853 1852 1851 1850 1844 1843 1842 1838 1836 1831 1830 1829 1825 1824 1817 1814 1813 1811 1808 1807 1804 1803 1802 1801 1800 1798 1794 1791 1789 1787 1783 1782 1781 1778 1775 1774 1772 1771 1766 1753 1751 1748 1735 1718 1700 1690 1687 1682 1671 1669 1624 1617 1520 1000 -300 -450 -800 -1000 -2000 -3500 -3750 -5000 -6000 -7000 -9000

Periodic Tables referencing the text string "Samanez", listed by date:

Orbital Filling With Electrons

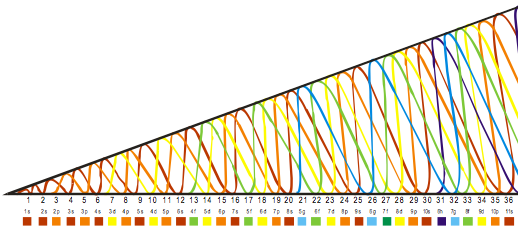
Students of chemistry are often confused why the orbitals fill with electrons: 1s², 2s², 2p⁶, 3s², 3p⁶, 4s², 3d¹⁰, 4p⁶... etc., because the 3d¹⁰ seems to be 'out of sequence'.

This 'out of sequence' difficulity is nicely explained if the orbitals are arranged in a slightly different way:

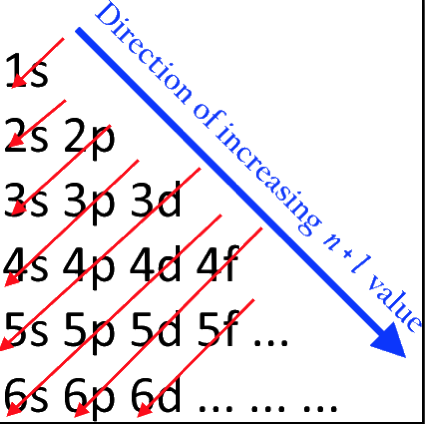
The aufbau principle states that in the ground state of an atom or ion, electrons fill atomic orbitals of the lowest available energy levels before occupying higher levels. For example, the 1s shell is filled before the 2s subshell is occupied. In this way, the electrons of an atom or ion form the most stable electron configuration possible.

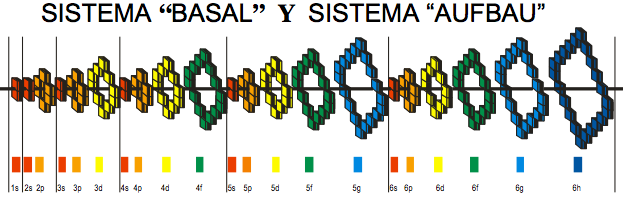
The order in which these orbitals are filled is given by the n + rule, also known as the Madelung rule (after Erwin Madelung), the Janet rule or the diagonal rule.

Orbitals with a lower n + value are filled before those with higher n + values. In this context, n represents the principal quantum number and ? the azimuthal quantum number. The values = 0, 1, 2, 3 correspond to the s, p, d and f orbital lables.

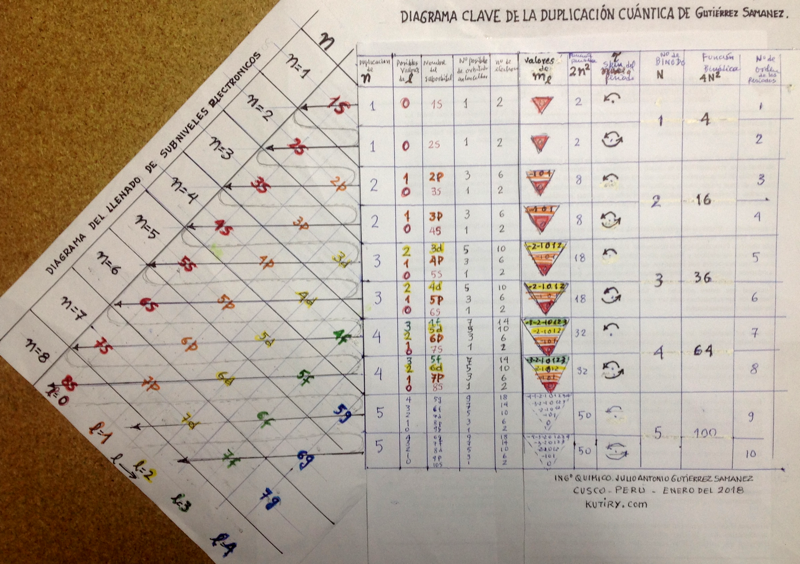
Julio Gutiérrez Samanez writes:

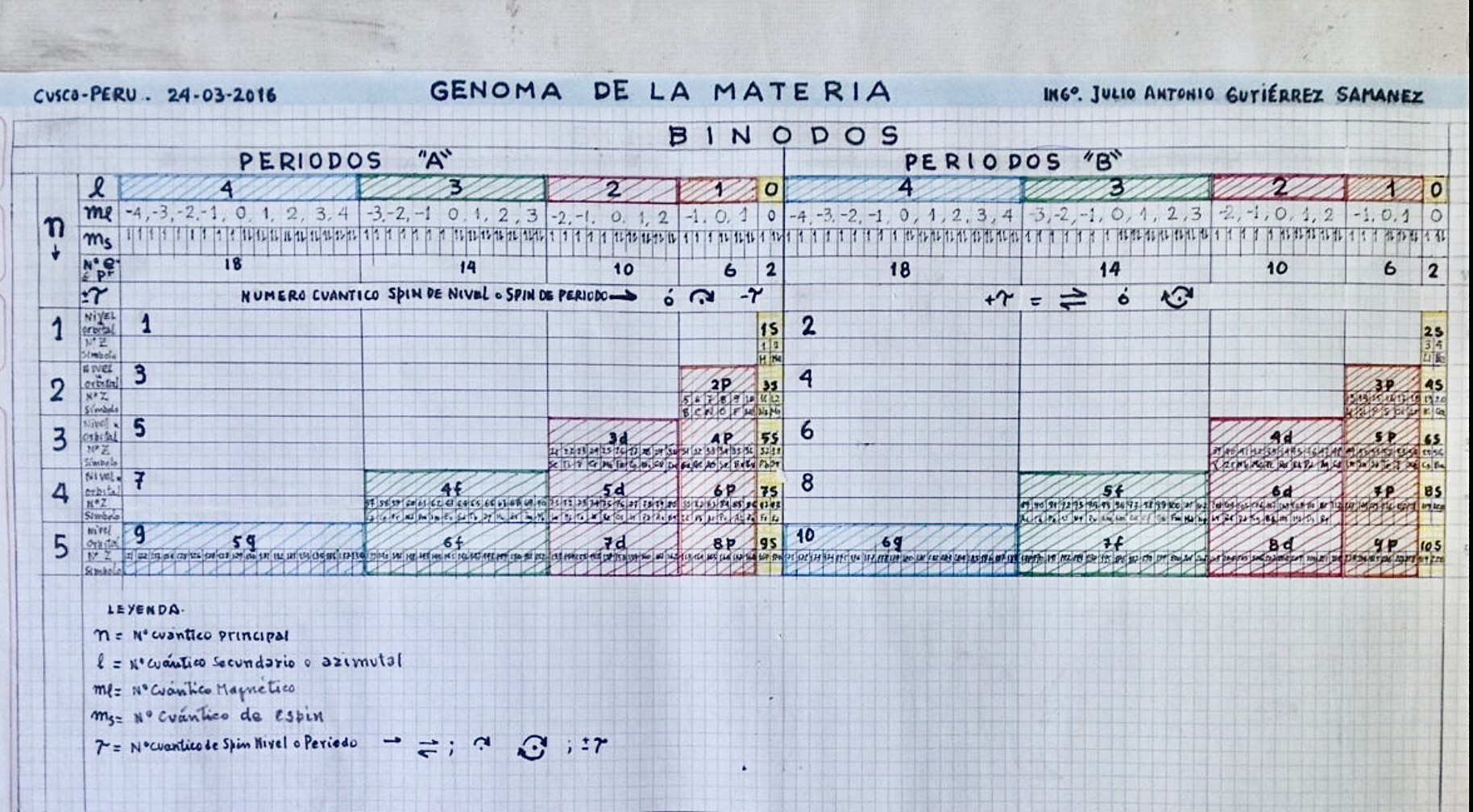
"I send you the diagram below that reconciles quantum mechanics (diagram for filling the electronic cells) with the Janet table or LSPT. Explaining the duplication of periods with the duplication of the quantum number n, and the introduction of Tao (T) spin of the level or spin of the period, which explains the parity of the symmetric periods."

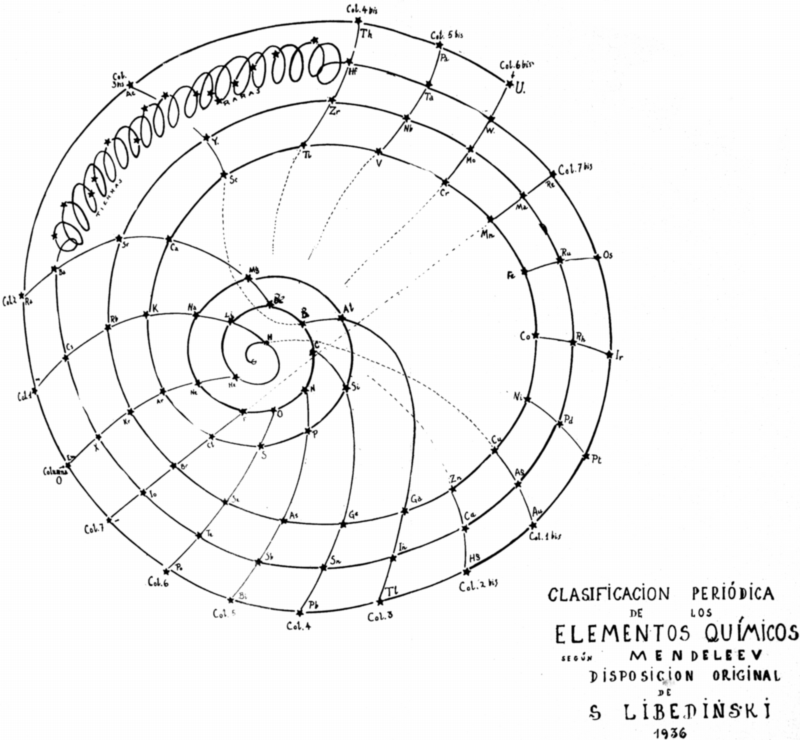
Libedinski's Periodic Classification of The Elements

Simón Libedinski: PERIODIC CLASSIFICATION OF THE ELEMENTS, from his book: Dialectical Materialism, in Nature, in Society and in Medicine, Ediciones Ercilla, Santiago de Chile, 1938, pp 56-57:

"Mendeleev's Table, like that of Werner and others, are not, however, more than flat projections of the actual ordering of the elements. There is as much difference between Mendeleev's Table and the real group as there is between the planisphere and a rotating globe. A rational representation, starting from the simplest element – the negative electron –, would be a spiral line that, surrounding said central point, first gave a small turn, touching only two bodies: hydrogen and helium. From here it would jump to a much larger orbit, in which it would touch eight bodies and then another equal, also of eight. From here, another jump to a much larger orbit, comprising eighteen bodies, and then another equal; from this point one jumps to another orbit, again augmented, comprising thirty-two bodies (including rare earths); and when this round is over, the last one begins, to vanish a short distance.

"In the dialectical grouping of the elements, which I have the satisfaction of exposing, the classic arrangement of the same is respected. Only the arrangement changes, which instead of being rectilinear, is spiral. So I managed to suppress the anomaly of the double columns, and comfortably incorporate the important group of rare earths. I can not give my graphic the name of Tabla, because it is just the opposite: it aims to give the idea of ??space, and of movement in space. The double columns of the Classic Table can be found here as well, but only if you look through the whole, considered as a planetary system of conical shape, with the electron at the vertex. Effectively: column 1 coincides, through space, with column 1a; column 4 with column 4 bis, etc. The dialectical grouping also allows us to easily appreciate the remarkable dialectical character of the properties of matter: these properties are repeated periodically. These are the "returns" to qualities or previous properties, but not exactly equal to those, but only similar: and this resemblance, only to a certain extent. The difference is that that quality, those properties or some characteristic, are exalted to each dialectical return."

Contributed by Julio Antonio Gutiérrez Samanez, Cusco, Peru, March 2018 (using Google Translation)

Mendoza's Neuvo Sistema Periodico

A memorial work, Ley De Configuraciones Electronicas, published posthumously in 1965 to honor Oswaldo Baca Mendoza (1908–1962 Cusco, Peru) and his 1959 Neuvo Sistema Periodico. Download the full PDF file (in Spanish).

Click to enlarge:

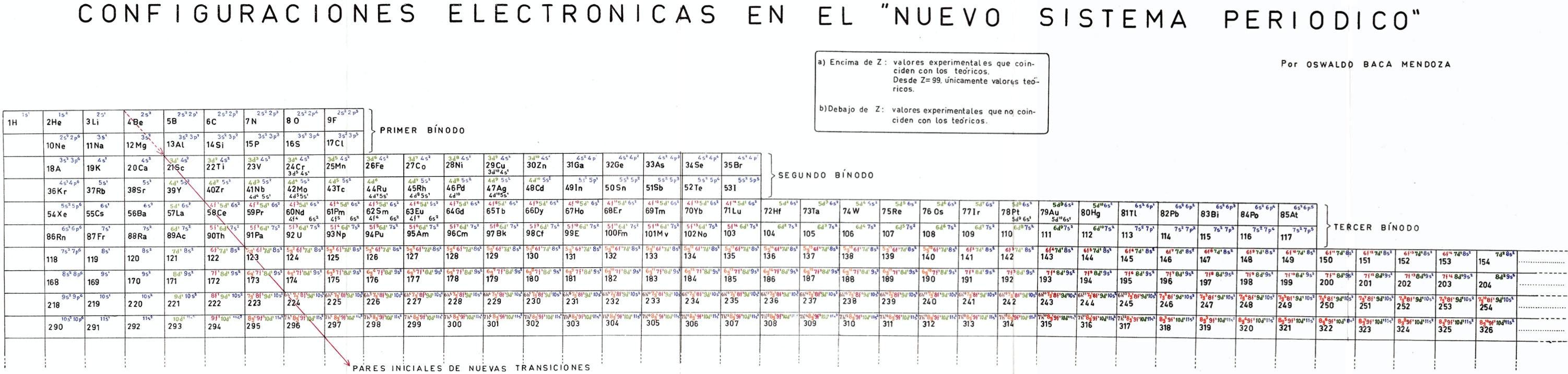
Thanks to Julio Gutierrez Samanez for the infomaton, etc.

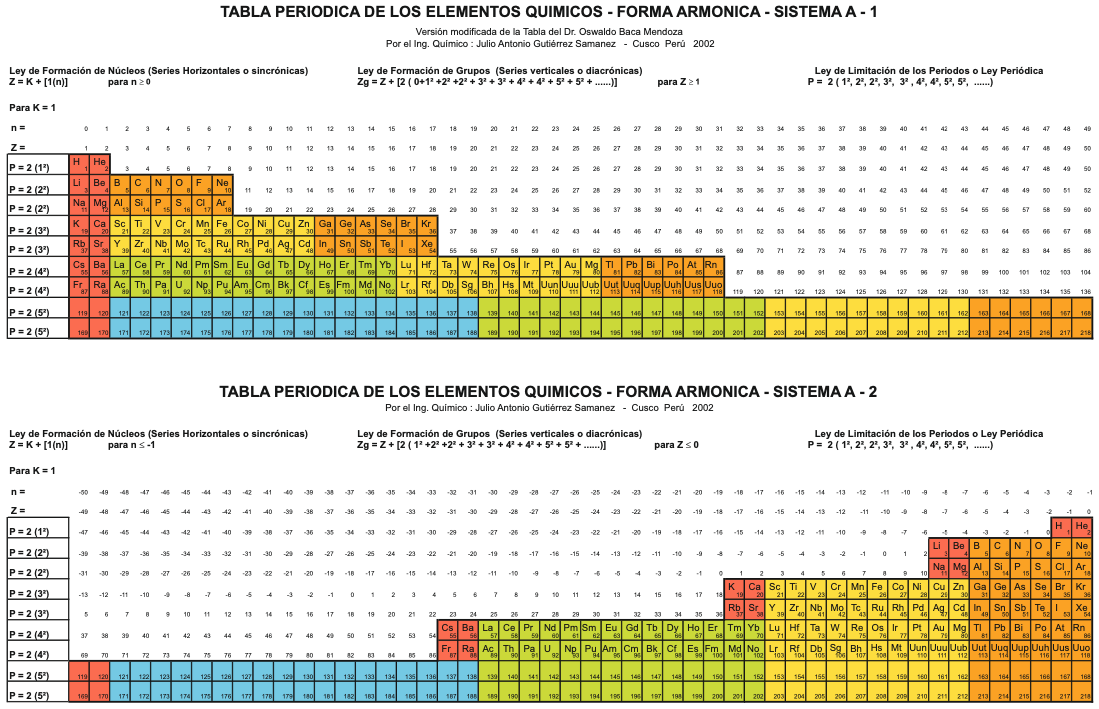
Sistema Periódico Armonico de Gutierrez-Samanez

A digitised 2004 book by book by Julio Gutiérrez-Samanez.

Julio writes:

"These matrix tables are inspired by the method used by the Peruvian chemist Oswaldo Baca Mendoza (1908-1962).

"The tables are read in this way: The Law of Formation of nuclei generates all the horizontal series Z, is dependent on n (series of integers numbers) and a constant K = 1. In the step-to-right tables (n) it will be equal to or greater than 0. In Janet's Left-Step table, (n) will be less than or equal to (-1). The values of this series Z will serve as a constant for the second law.

"The Group Formation Law or vertical series. Its generate the numeric values of the columns either from left to right or from right to left. In system A -1: With the first law: n = 0, then Z = 1. The vertical series Zg = 1, 3 11, 19, 37, 55, 87 ... That is: 1H, 3Li, 11Na, 19K, 37Rb, 55Cs, 87Fr, 119, 169 ... Changing the values of n or Z, all the columns of the table will be obtained.

"In system A -2: With the first law: on the left, n = -1, then Z = 0. The vertical series Zg will be: 2He, 10Ne, 18Ar, 36Kr, 54Xe, 86Rn, 118Og, 168, 218. .. Similarly, changing the n or Z values, we can fills the columns of the table. In system B -1: With the first law: for n = 0, then Z = 1. The vertical series Zg will be; 1H, 3Li, 5B, 13Al, 21Sc, 39Y, 57La, 89Ac, 121, 171 ... By varying the values of n or Z, the entire table is filled. In system B -2: (Its mathematizes the Janet system). With the first law: on the left, for n = -1, then Z = 0.

"The vertical series Zg will be; 2He, 4Be, 12Mg, 20Ca, 38Sr, 56Ba, 88Ra, 120, 170, 220 ... By varying the values of n or Z, the entire table is filled. The third law of the limiting the periods or periodic law, appears graphically, by comparison between rows: For example: in table B -1, in column Z = 3, after 1H and 2He, en of the first horizontal line, the value 3 appears, which is already entered in the first column as 3Li, therefore, that part of the first horizontal row (from 3 to 50) is deleted.

"The same happens with the number 5 in column 3, which is already in the first column as 5B, therefore it will be deleted in the second row from 5 to 52. The same applies to pair 13, 21 of the column Z = 9, same, with the pair 39, 57 of the column Z = 19 and of the pair 89, 121 of the column Z = 33. For that reason the periods: P are duplicated function: 2 (1 ^ 2), 2 (1 ^ 2), 2 (2 ^ 2), 2 (2 ^ 2), 2 (3 ^ 2), 2 (3 ^ 2) .... = 2, 2, 8, 8, 18, 18, 32, 32 ... and the forms are exact and staggered. The colors represent the quantum functions: s (red), p (orange), d (yellow), f (green), g (blue)."

Read the book here (in Spanish).

Nuevo Modelo Mathemático Tabla Periódica

Julio Antonio Gutiérrez Samanez presents his Periodic Table formulation ideas in a 2006 PDF paper (in Spanish):

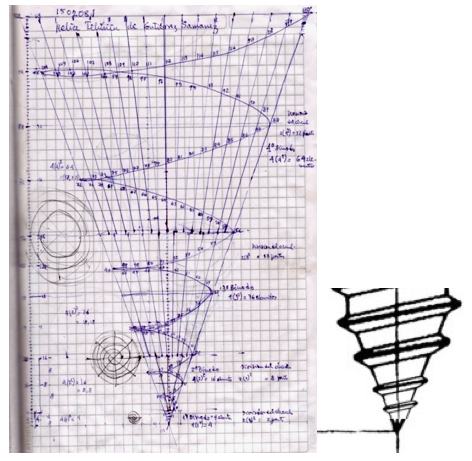
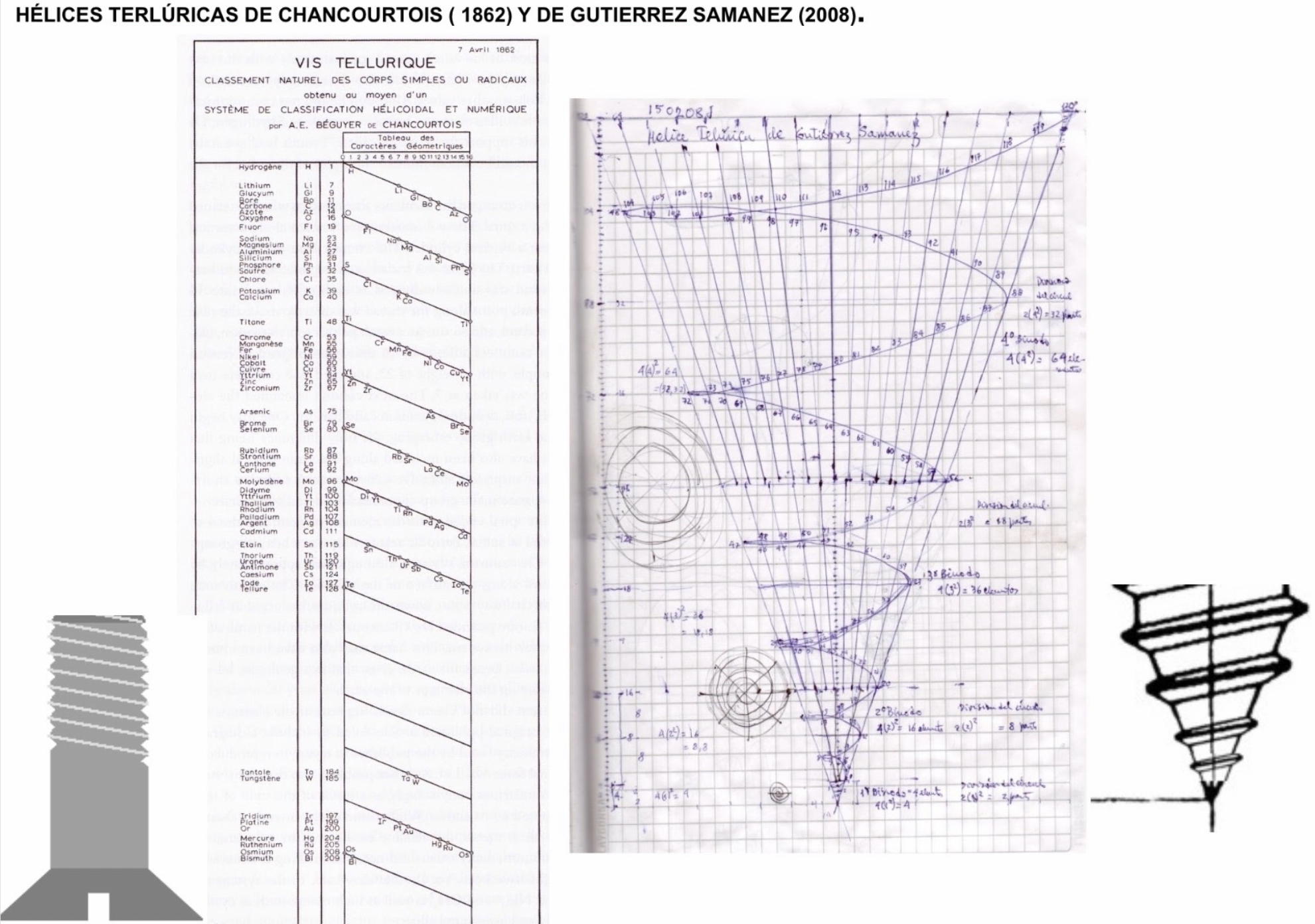
Teluric Helix from Gutierrez Samanez

The Teluric Helix from Gutierrez Samanez is inspired by the telluric helix Chancortois (1864) with the difference that the sequence of the elements are rolled into a cone shape rather than a cylinder:

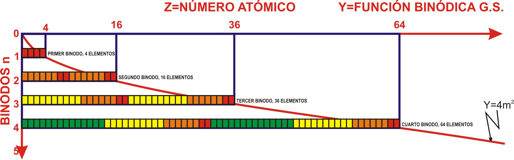
Khipu or Quipu Periodic Table

The Khipu or Quipu or Talking Knot Periodic Table, developed by Julio Antonio Gutierrez Samanez.

Google translated from the Spanish pdf file:

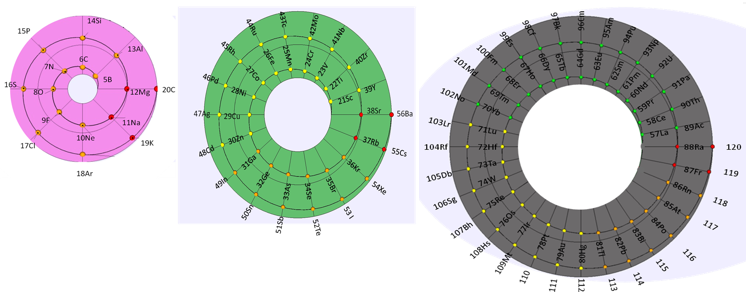
"As a result of bringing together each pair of periods in a single function or binod, the author has found a new regular on the subject, which has been defined as a new quantum number, since the number of orders or regulations binod growth elements in the table, under the appearance of pairs of new types of quantum structures or periods whose organization responds to a simple mathematical function: a parable of the type Y = 4 X ^ 2 - In this case report: a) That the strings correspond to pairs of periods or binod and knots are double for items with orbital s (in red), six nodes for p in orange, 10 yellow d knots and 14 knots for green f . b) That in each binod or rope, appear regularly in pairing mode or dual, new quantum or orbital structures, such as moving from within the orbital previous binod.":

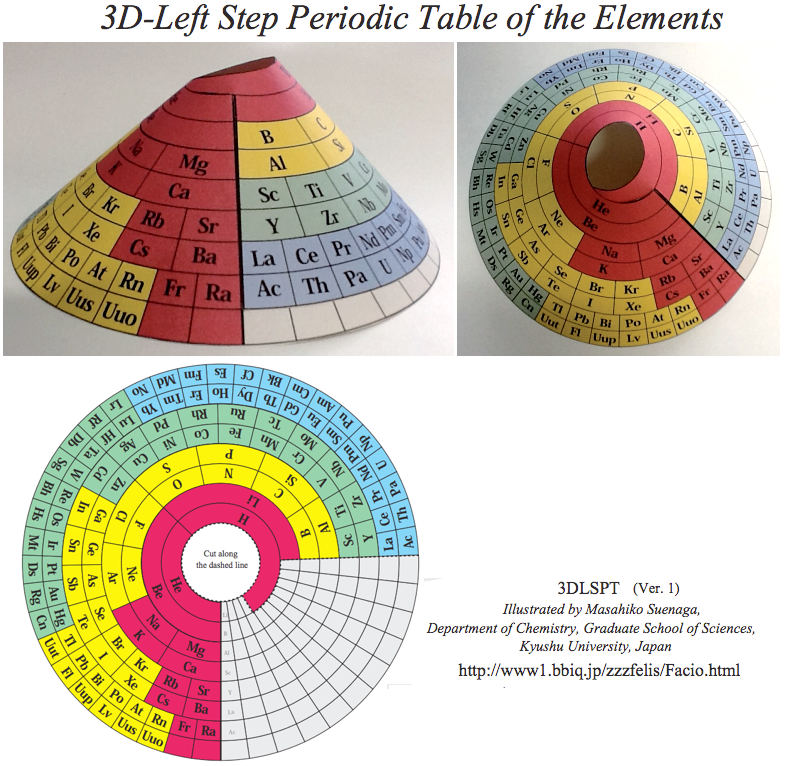
3D Left Step Periodic Table

By Masahiko Suenaga, Kyushu University, Japan a 3D Left Step Periodic Table.

"Inspired by the work of Dr. Tsimmerman and Dr. Samanez, I have created a new 3D Left Step Periodic Table, which resembles to Mt. Fuji, recently registered as a World Heritage site. For more information, please visit my website":

Gutierrez Samanez's Binodic Form of the Periodic Table (Video)

Genoma de la Materia

By Julio Antonio Gutiérrez Samanez, Genome of Matter:

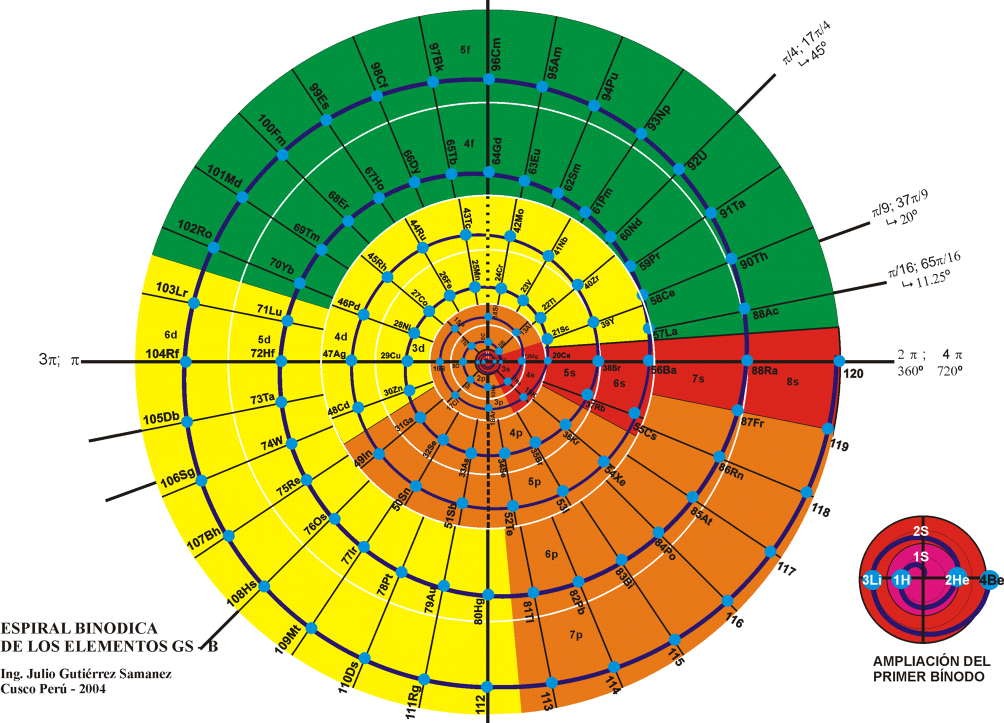
Sistema Periódico Binodico

By Julio Antonio Gutiérrez Samanez, who writes:

"Sistema Periódico Binodico. Nuevo Paradigma Matematizado. I have followed the work of the wise Mendeleev, of Emil de Chancourtois, of Charles Janet; inspired by the work of my countryman Dr. Oswaldo Baca Mendoza. It is in Spanish but soon I will have the English version."

Periodical System (Binodic Form): a new mathematical paradigm

By Julio Antonio Gutiérrez Samanez, who writes:

"System devised and prepared by the Peruvian chemical engineer, Julio Antonio Gutiérrez Samanez, deals with a new conception of Mendeleev's Law as a mathematical function and a new description of the process of forming the series of chemical elements according to mathematical laws and dialectical processes of changes quantitative and qualitative under a dynamic spiral architecture in 3D, which is postulated as a new scientific paradigm."

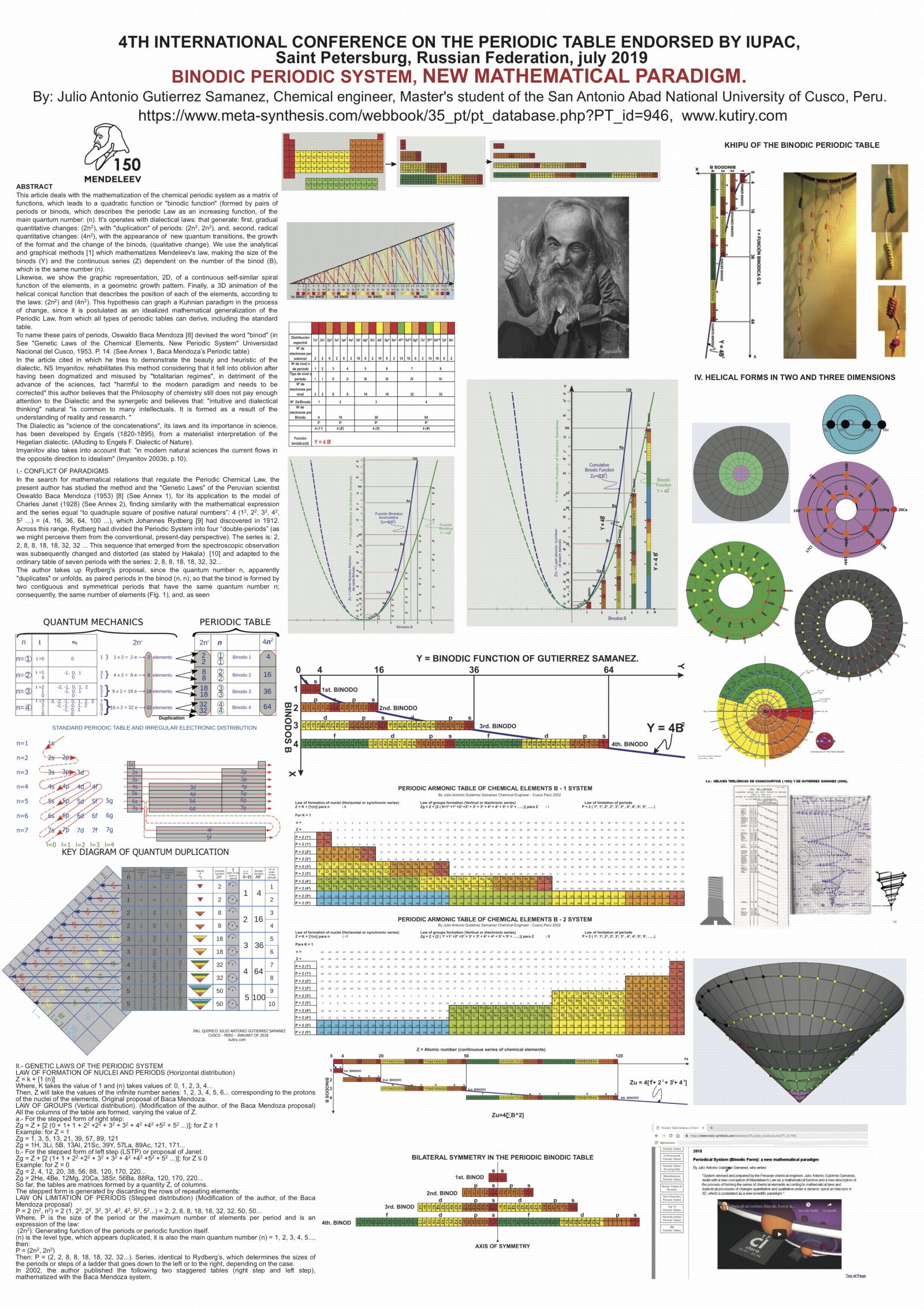
Samanez's Binodic Periodic System, New Mathematical Paradigm Poster

Julio Antonio Gutierrez Samanez (Master's student in chemical engineering at the San Antonio Abad National University of Cusco, Peru) presented a poster at the 4th International Conference on the Periodic Table arranged by IUPAC, Saint Petersburg, Russian Federation, July 2019. See the high resolution .PDF file.

More here and at kutiry.com        

Helix vs. Screw

Julio Antonio Gutierrez Samanez writes:

Until today, when they write about the work of Chancourtois and his telluric helix wound in a cylinder, still no one alludes to this other telluric helix wound in a cone or screw, the idea is the same: a rope that winds a geometric solid.

The first was devised in 1862, the other in 2008 (146 years later). But, there is a big epistemological difference. In the first, the elementary series presented was: 8, 8, 8, 8, 8 ..., etc., in the second it is: 2, 2, 8, 8, 18, 18, 32, 32. Furthermore, the division of conical radii is regulated by the function 2 (n ^ 2) = 2, 8, 18, 32...

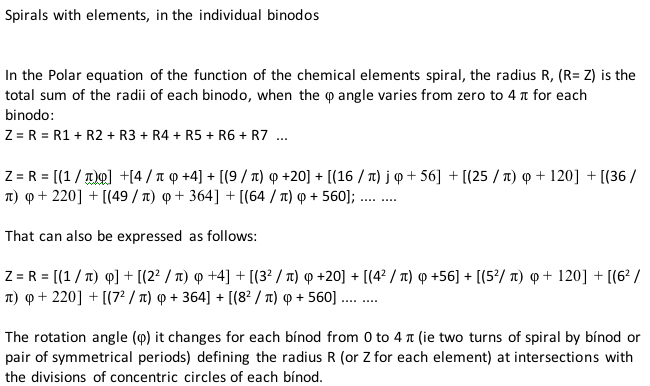
Each binode has two spirals or two periods with the same number of elements, which correspond to the function 4 (n ^ 2). I don't think it is a discovery, it is just the conclusion of the contributions of Rydberg, Janet, and, of course, Chancourtois.

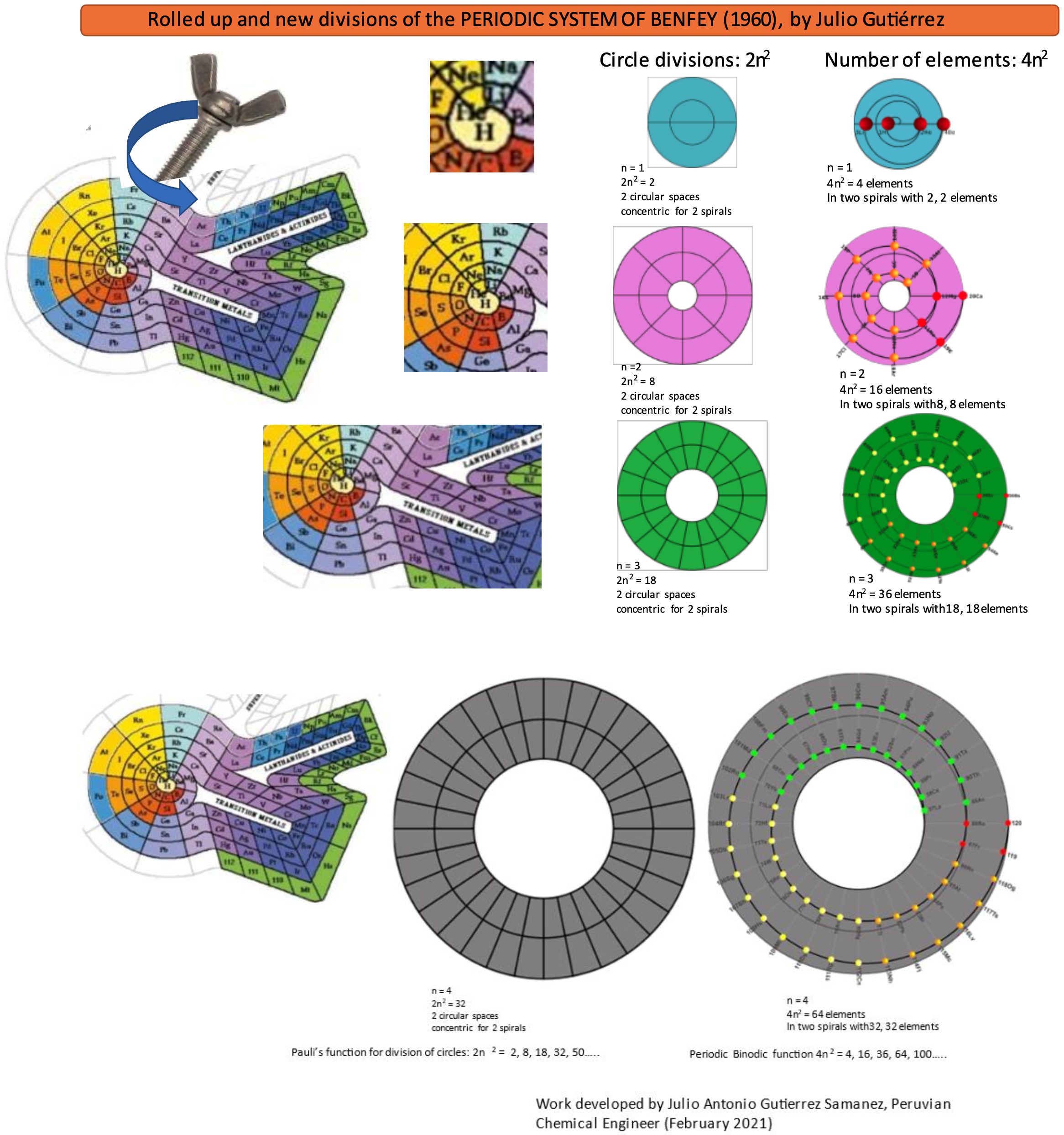
Rolled-up Version of Benfey's Periodic System

Rolled-up Version of Benfey's Periodic System by Julio Antonio Gutiérrez Samanez. More on the YouTube video here.

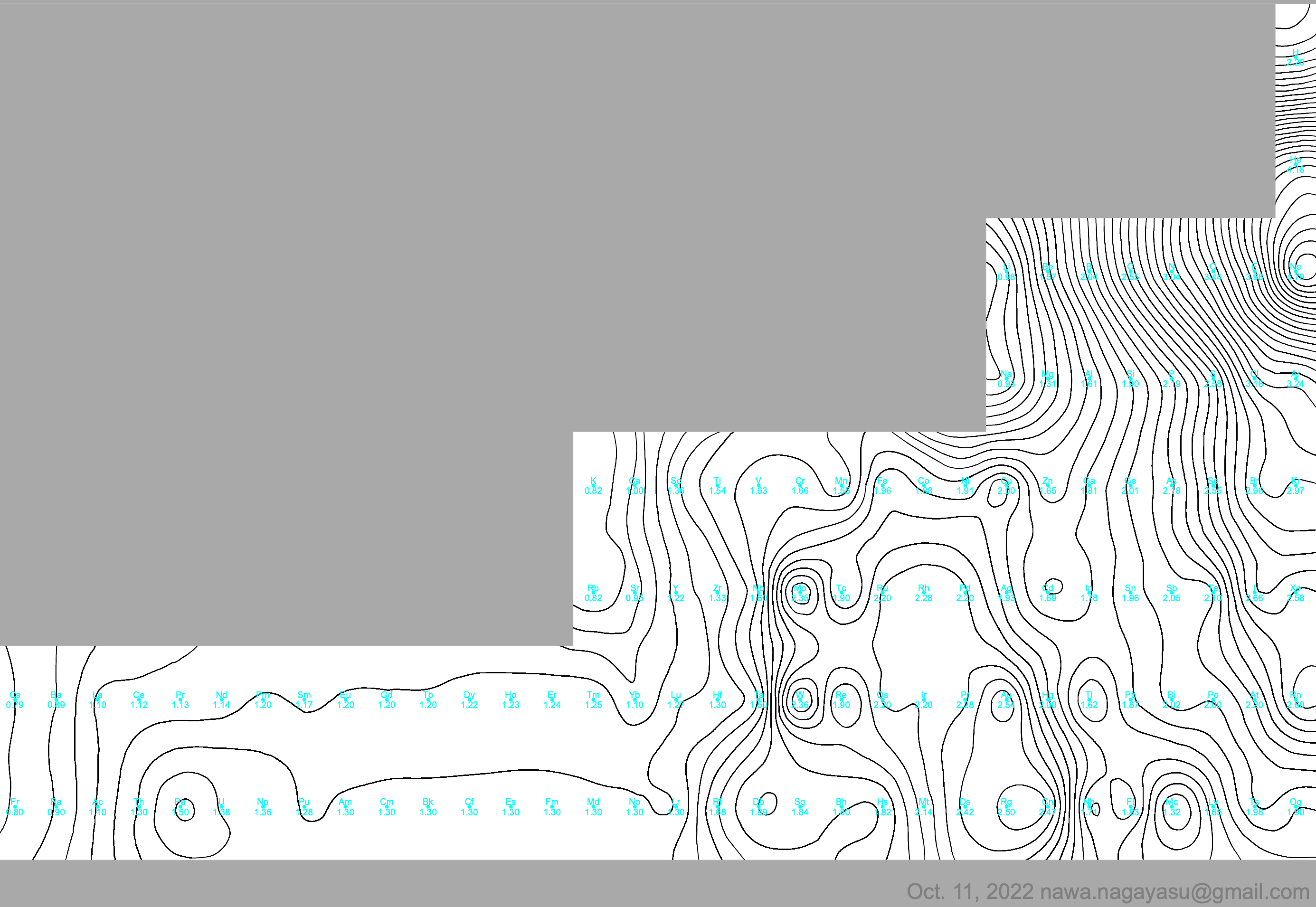
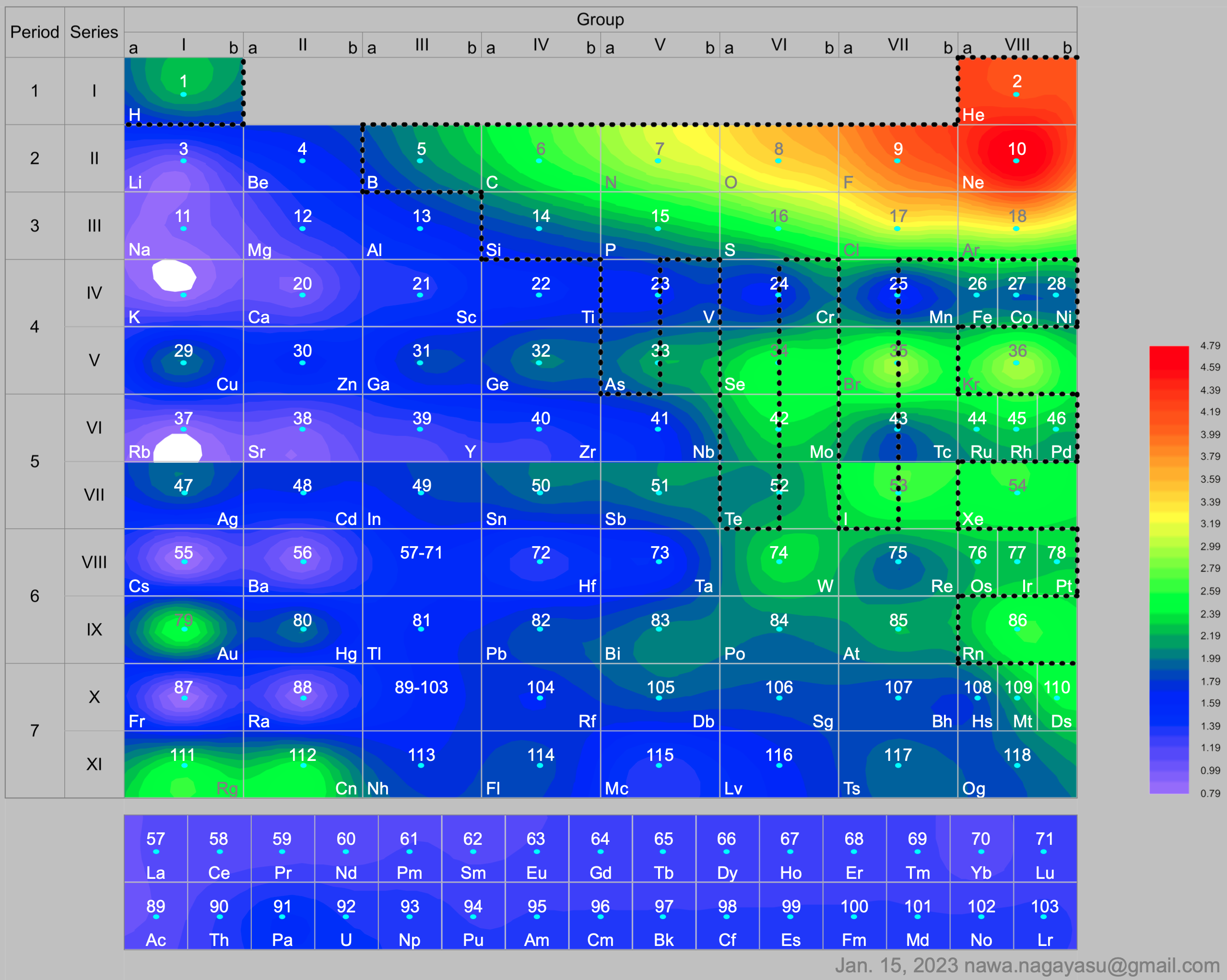
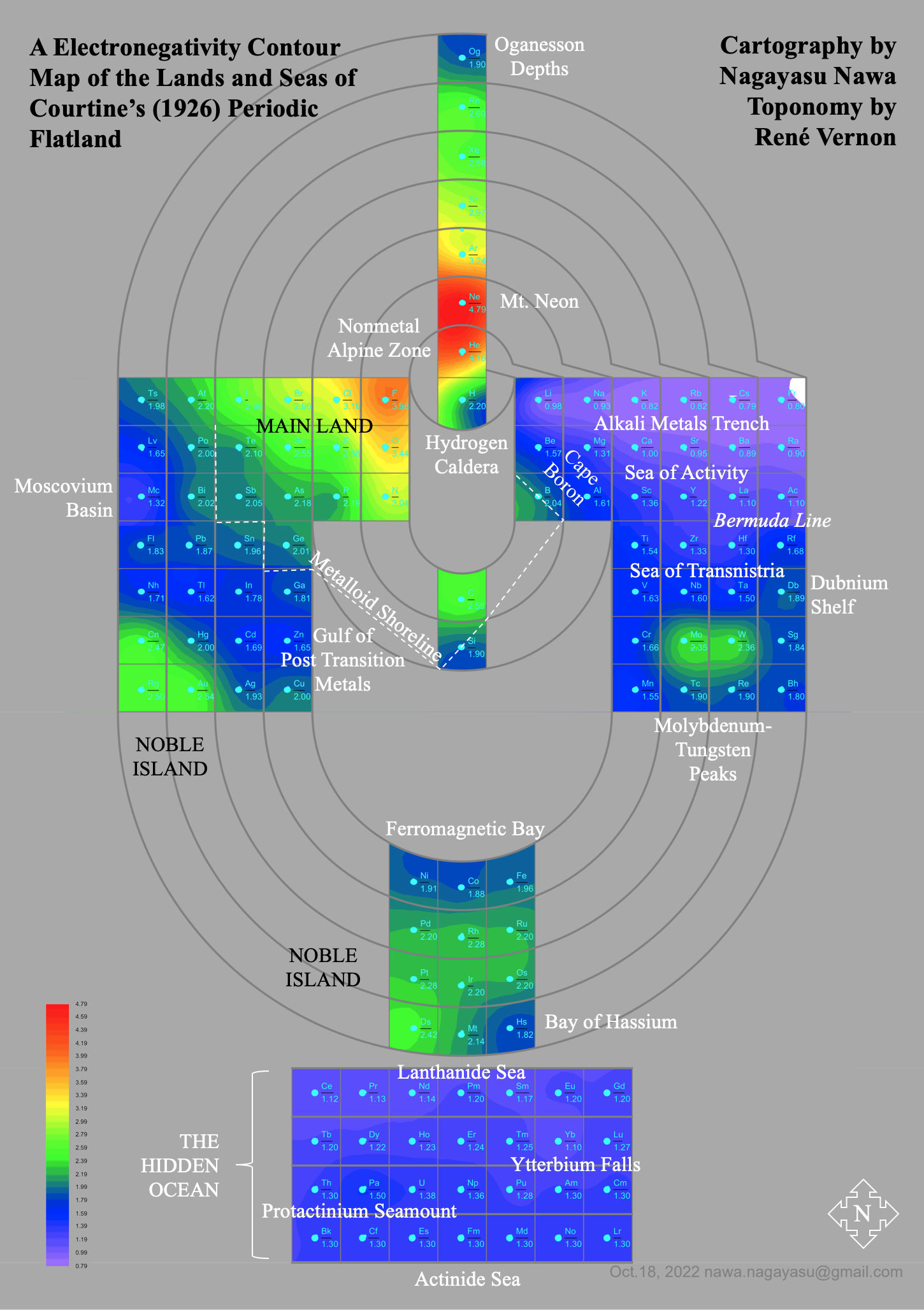
Electronegativity Seamlessly Mapped Onto Various Formulations of The Periodic Table

A discussion on the Google Groups Periodic Table Discussion List, involving a René Vernon, Nawa Nagayasu & Julio Samanez (all contributors this database) lead to the development of the representations below, showing electronegativity seamlessly mapped onto a modified Left-Step Periodic Table:

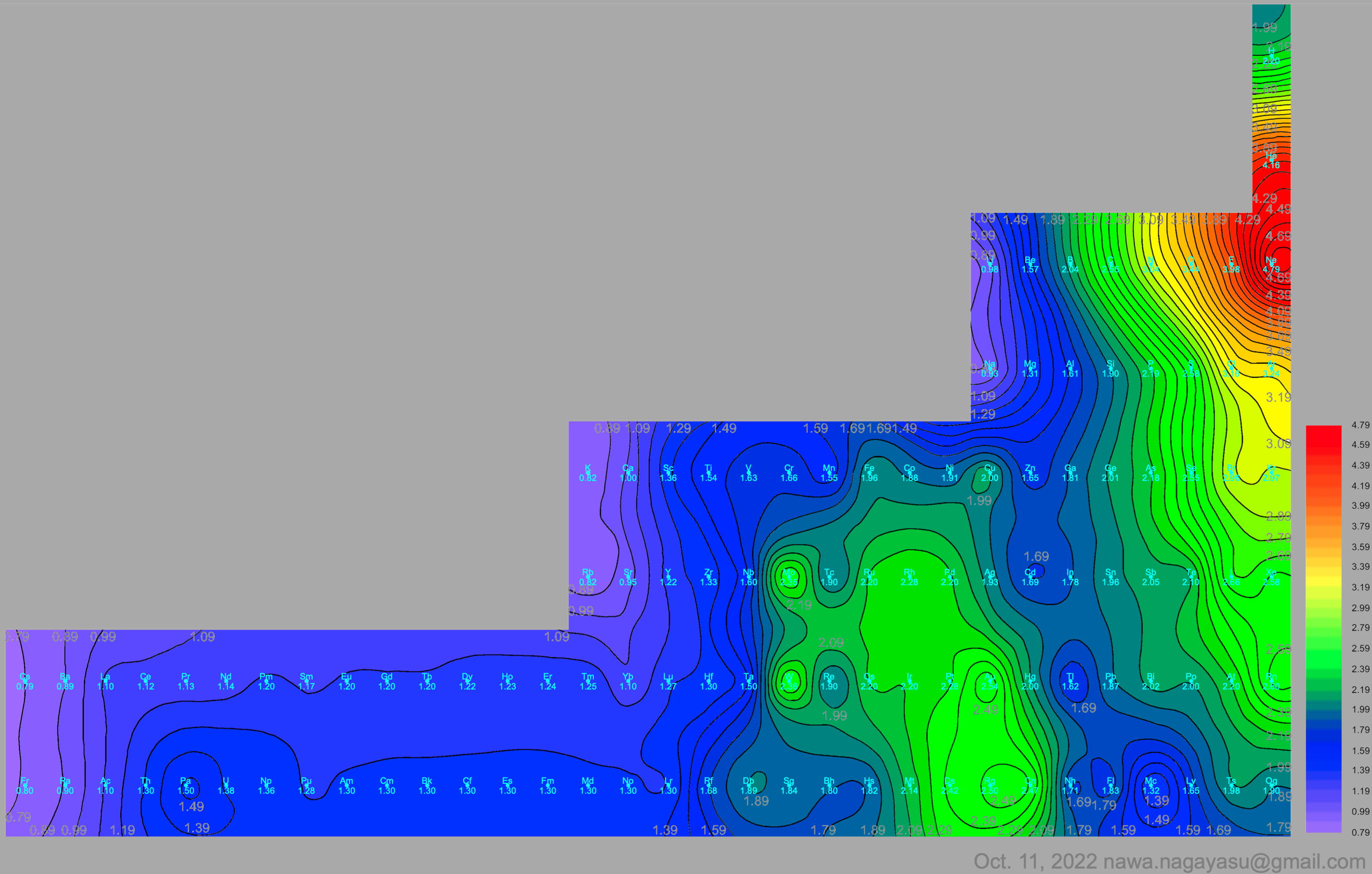
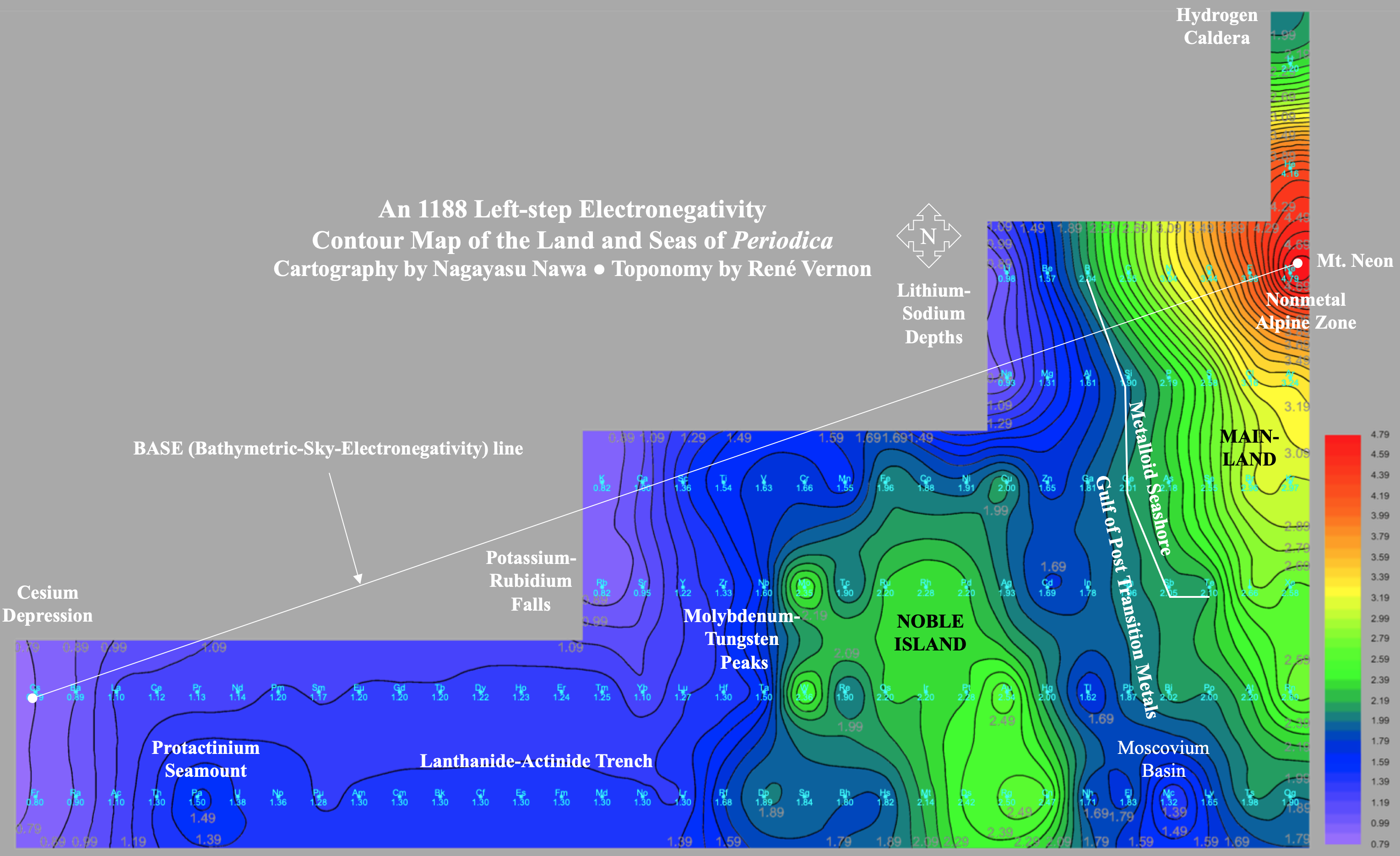
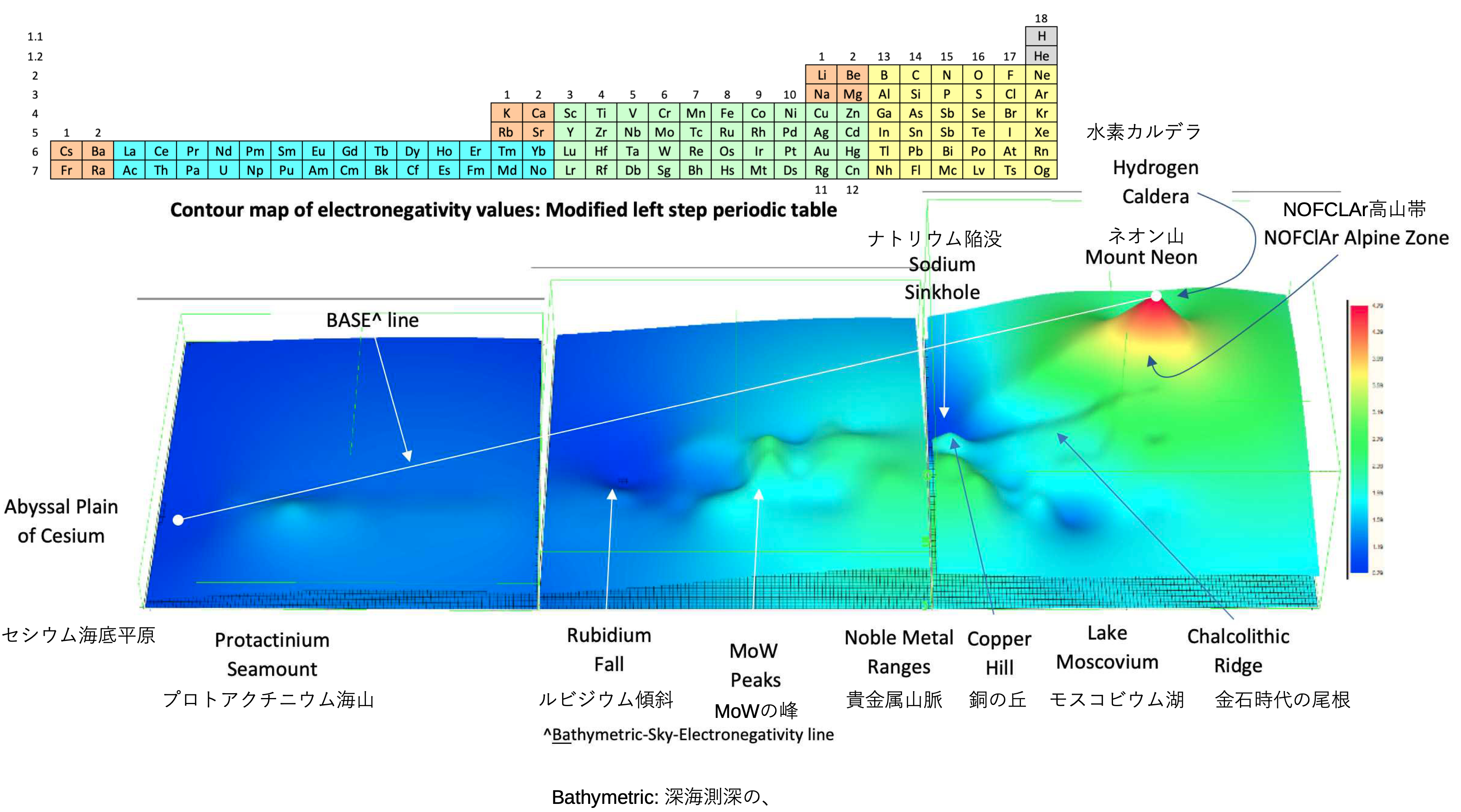
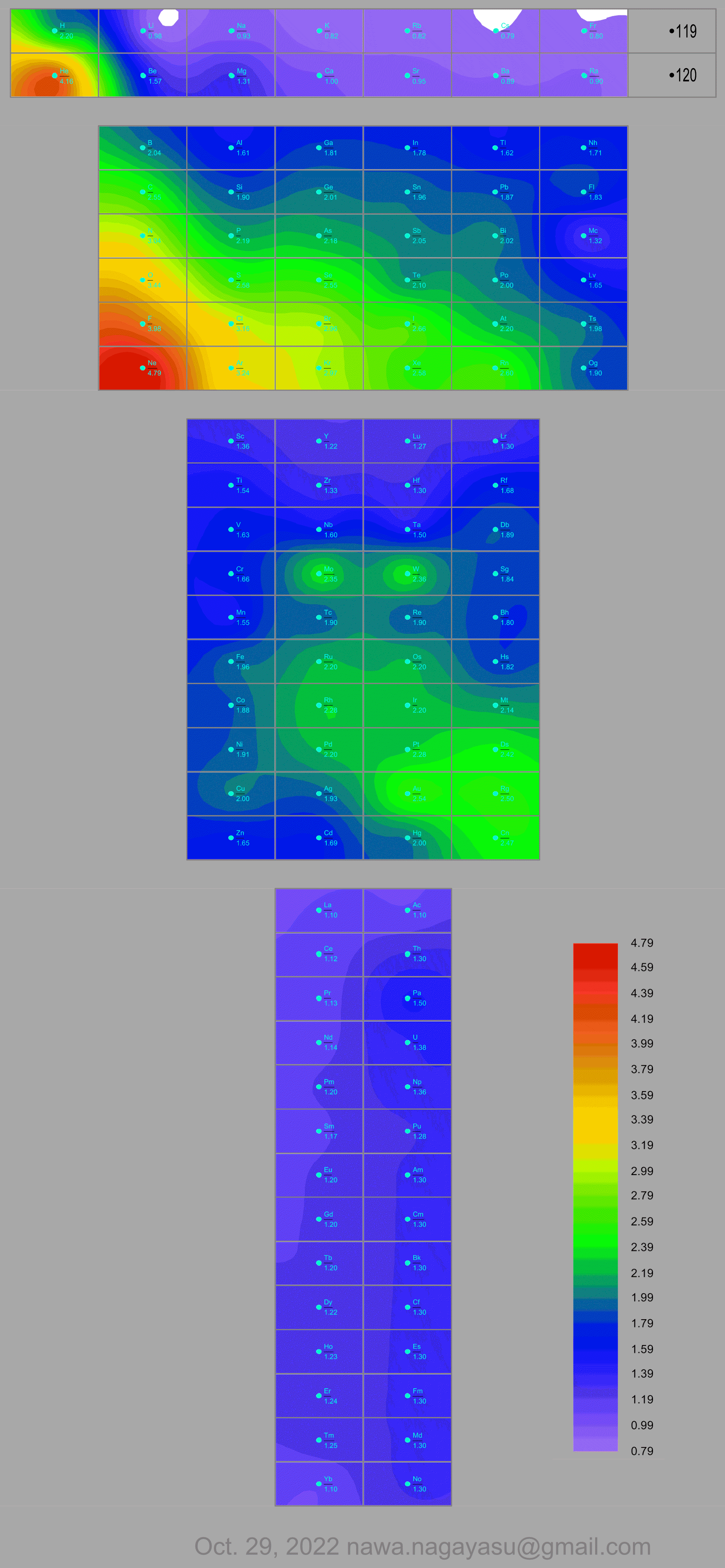
Nawa Nagayasu has mapped electronegativity to Mendeleeve's formulation:

Nawa Nagayasu has mapped electronegativity onto other formulations, Julio's Binode Spiral:

Courtine's 1926 formulation:

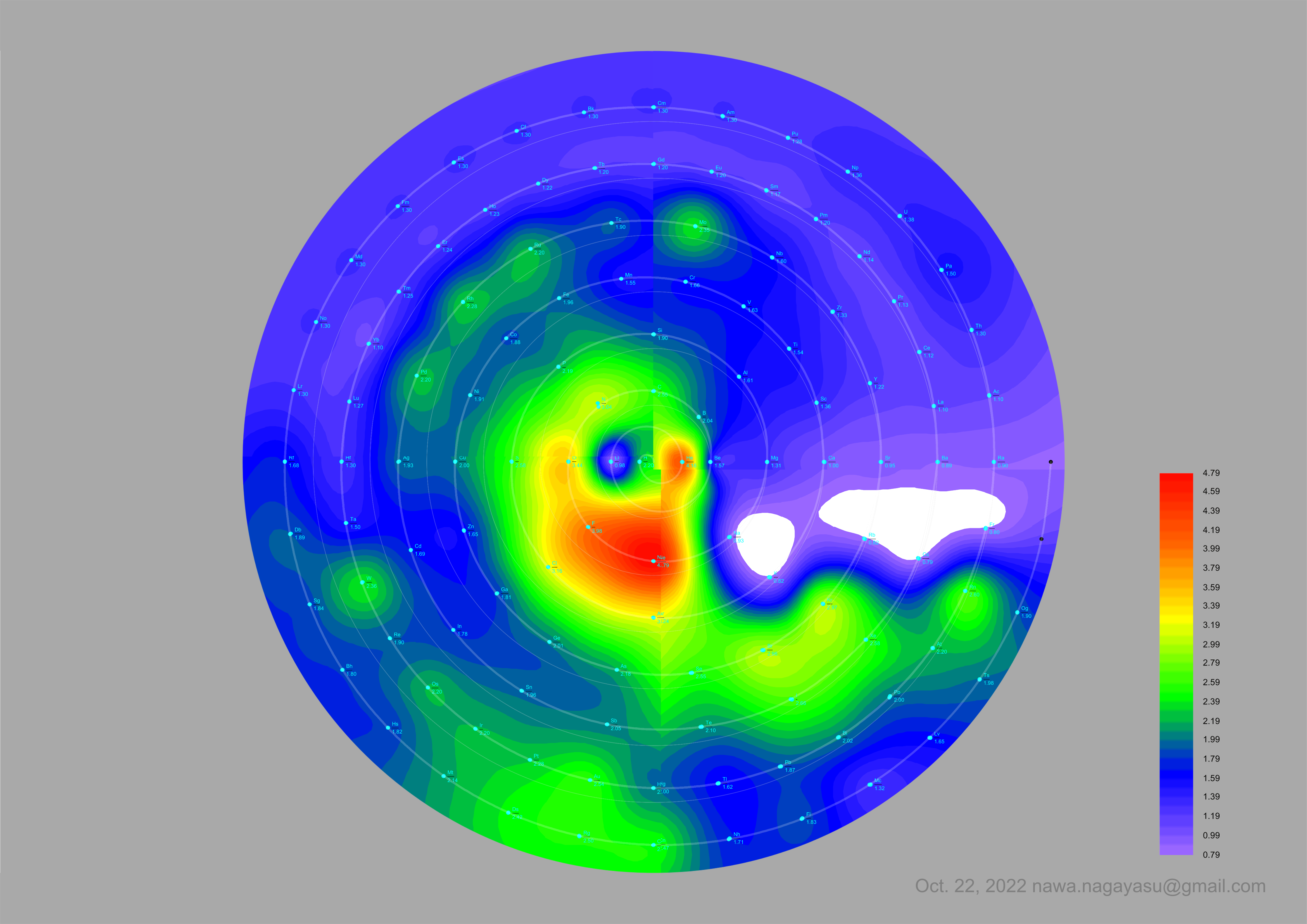
and the "conventional", short, medium and long forms of the periodic table with hydrogen above and between B & C which show the botom-right-to-top-left electronegativity trend:

Jeff Moran's Spiral:

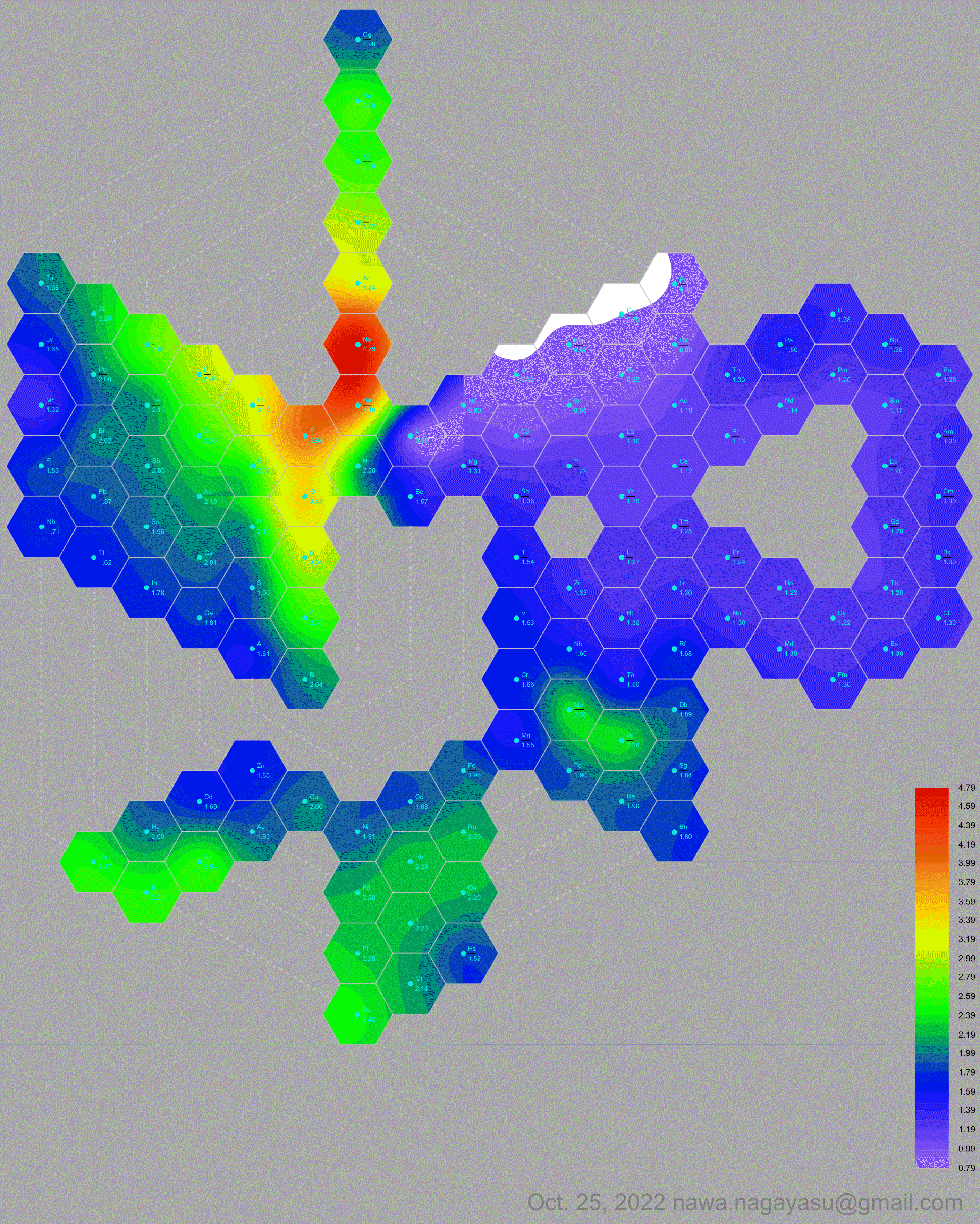
René Vernon's 777 Periodic Wedding Cake:

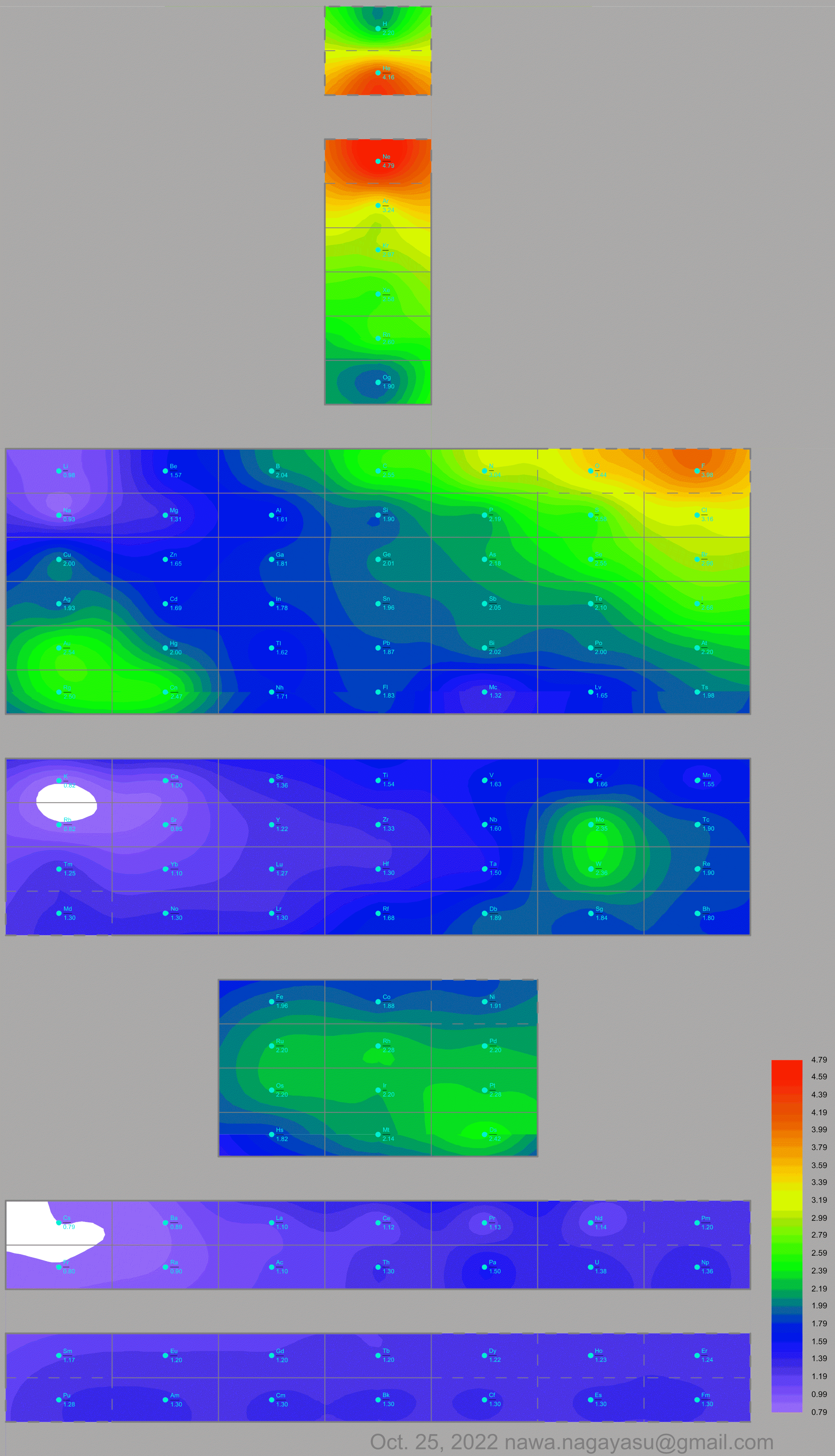
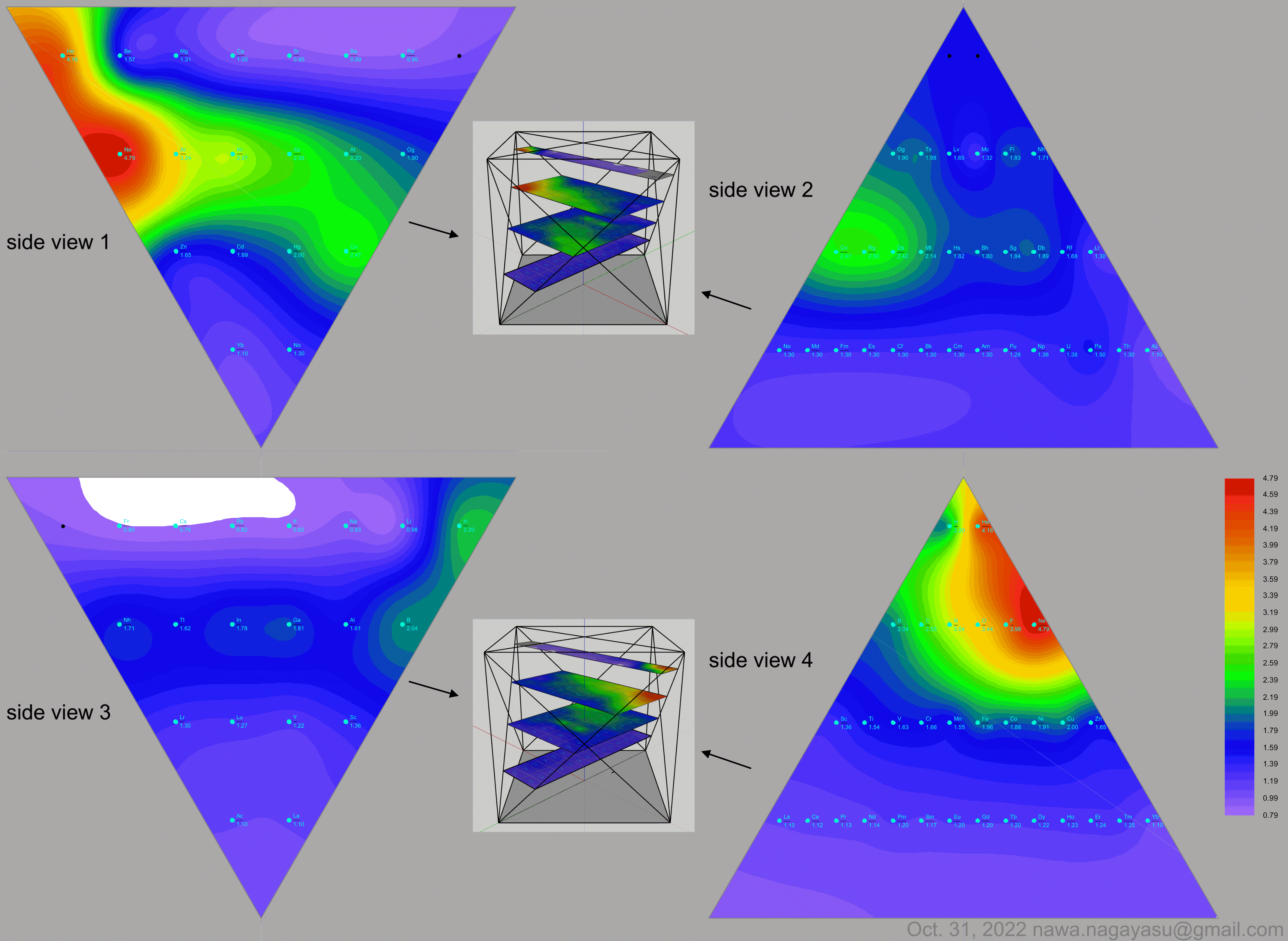
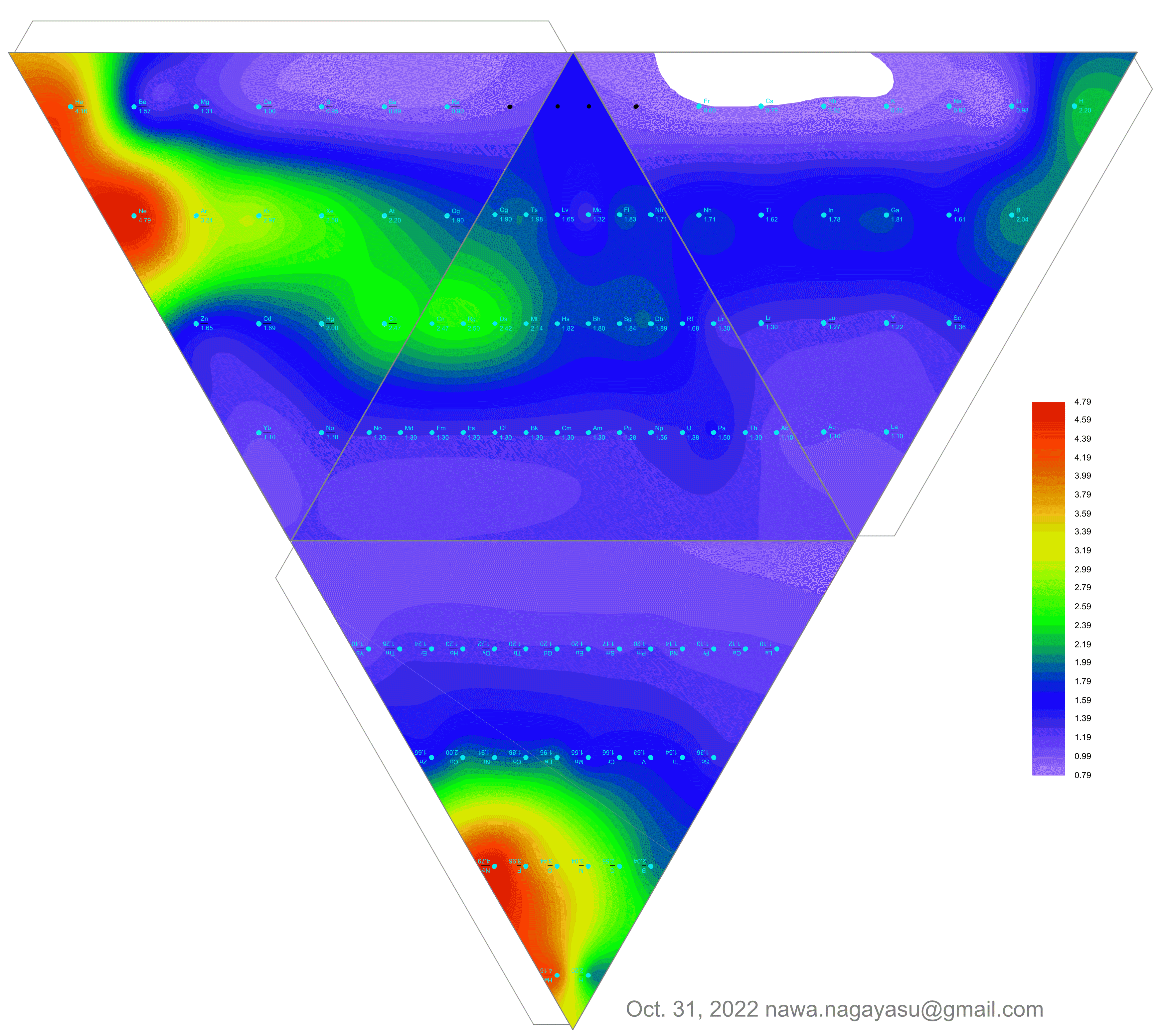
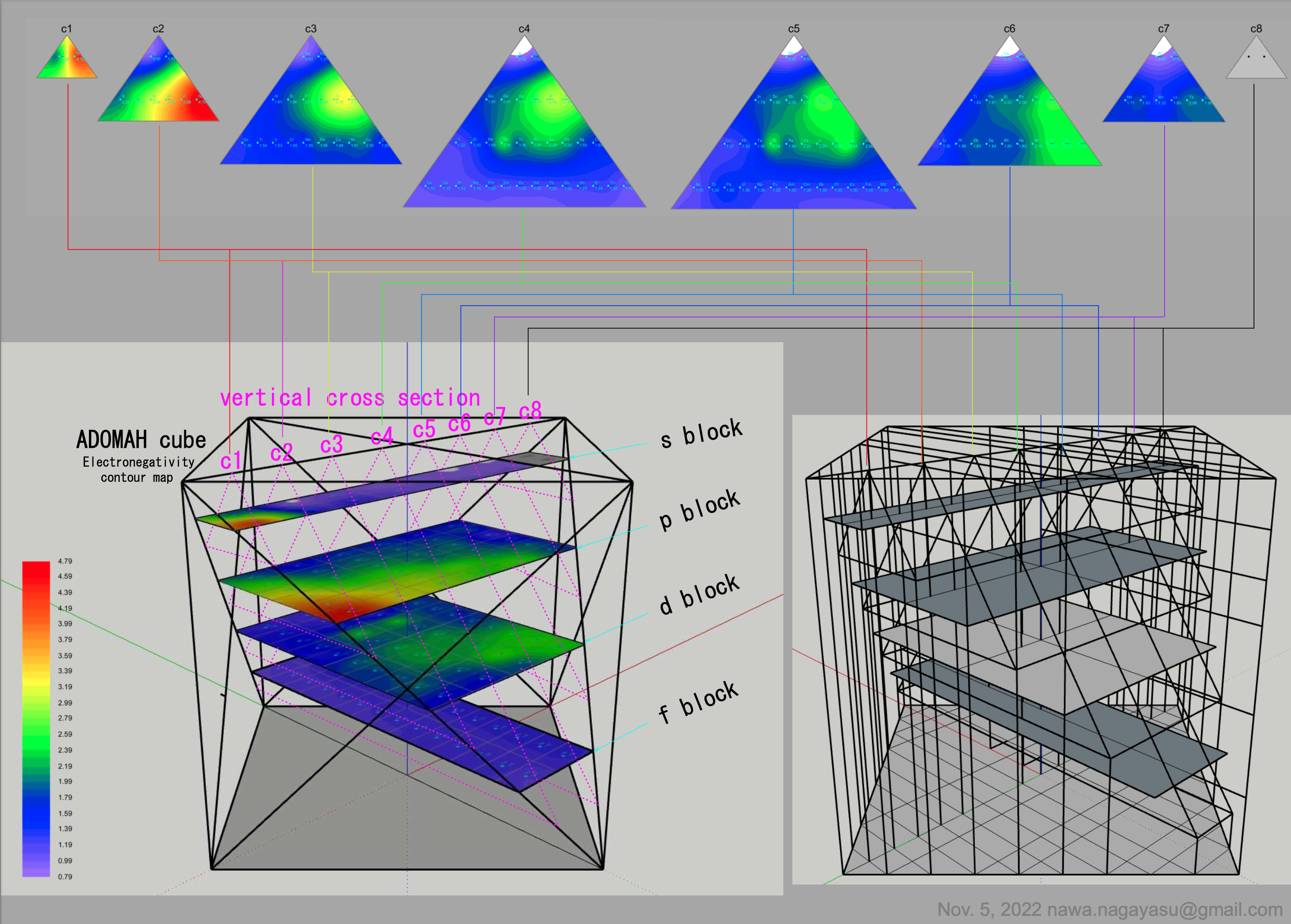
Valery Tsimmerman's ADOMAH formulation:

Valery Tsimmerman's ADOMAH tetrahedron (in a glass cube) formulation:

Bilateral Symmetry in the Periodic Binodic Table

René Vernon, who developed these ideas, writes:

This table is adapted from the work of Gutiérrez-Samanez (2020), who discusses mathematising the chemical periodic system as a grid, which leads to a quadratic function or “binódica function” formed by pairs of periods or binodos (dyads).

The difference is that whereas Gutiérrez-Samanez showed the first pair of periods as H-He and Li-Be, this table shows the first period as e-n and H-He. Here, e is the electron and n is the neutron. Each pair of periods is shown pancake style rather than in a single row. The formula for the length of each paired period or binode is 2n² = 2, 8, 16, 32.

The idea of paired periods has a long history; it seems to have originated with Werner in 1905.

According to Jensen:

"The temptation to read more into the shape of the table than is really there is almost overwhelming. Even someone as great as Werner was tempted (1905). Having postulated a missing element between H and He, he decided to perfect the symmetry of his table by guaranteeing that rows of differing length always occurred in pairs. Consequently, he further postulated a row of three missing elements lying above the H-X-He row."

Rydberg (1913, pp. 12–13) used a formula 4n^2 for the number of elements in the paired periods: 4, 16, 32, 64. This formula is also used by Gutiérrez-Samanez.

Paired periods were also used by Janet (1928), Saz (1931), Achimov (1946) and Baca Mendoza (1953).

References

• Achimov EI 1946, Zhur. Obshchei Khim., vol. 16, p. 961; https://www.meta-synthesis.com/webbook/35_pt/pt_database.php?PT_id=776
• Baca Mendoza O 1953, Leyes Genéticas de los elementos Químicos, Nuevo Sistema Periódico, National University of Cusco, https://www.meta-synthesis.com/webbook/35_pt/Mendoza_PT.pdf, accessed May 12, 2024
• Gutiérrez-Samanez JA 2020, Binódic periodic system: a mathematical approach, Found Chem, vol. 22, pp. 235–266 (255)
• Janet C1928, Essais de classification hélicoïdale des éléments chimiques. Imprimerie Départementale de l’Oise, Beauvais, https://www.meta-synthesis.com/webbook/35_pt/pt_database.php?PT_id=152
• Jensen WB 1986, Classification, symmetry and the periodic table, Computers & Mathematics with Applications, vol. 12B, no. 12, pp. 487–510 (508)
• Rydberg JR 1913, Untersuchungen über das system der grundstoffe, Lunds Univ. Ärsskrift, vol. 9, no. 18. In French: 1914, Recherches sur le système des éléments, Journal de Chimie Physique, vol. 12, p. 585
• Saz E 1931, Iberica, vol. 35, p. 186
• Wemer A 1905, Beitrag zum Aufbau des periodischen Systems, Ber. Deut. Chem. Ges, vol. 38, pp. 914–921, 2022–2027

© Mark R. Leach Ph.D. 1999 –

Queries, Suggestions, Bugs, Errors, Typos...

If you have any:

Queries
Comments
Suggestions
Suggestions for links
Bug, typo or grammatical error reports about this page,

please contact Mark R. Leach, the author, using mark@meta-synthesis.com

This free, open access web book is an ongoing project and your input is appreciated.